Relationship between Rebound Velocity and Ramp Height in a Billiard Ball
Background .:. Method .:. Results and Data .:. Conclusion .:. Sources .:. Links .:. Return to Research
Rose Newberry and Miranda Steed
Background: The most common use of a billiard ball today is for playing billiards or pool. Typically, billiard balls are made of polyester and clear acrylic[1]. Collisions between billiard are nearly elastic[2]. Physics often uses billiard balls to demonstrate Newton’s Laws of Motion because they roll on a surface that has low rolling friction[3]. Our experiment demonstrates Newton’s Third Law of Motion. Newton’s Third Law of Motion states that “when a first body exerts a force F1 on a second body, the second body simultaneously exerts a force F2 = -F1 on the first body. F1 and F2 are equal in magnitude and opposite in direction”[4].
Since there are so many different variables involved with billiard balls, we limited our research to those topics involving ramp height and its effect on rebound velocity. Most sources indicated that the higher the ramp the greater the rebound velocity[5][6]. We will use Newton’s Third Law of Motion, which is stated above. We will calculate the rebound velocity by dividing the distance travelled by the ball after colliding with the end of the pool table by the time it took for the ball to stop after colliding with the pool table. The initial velocity will be uniform for each trial within a certain height because the potential energy will be the same[7].
Statement of Problem: The purpose of this investigation is to find out the relationship, if any, between the height of a ramp and the rebound velocity of a billiard ball rolled down the ramp.
Hypothesis: We believe that rebound velocity will gradually increase with ramp height in our experiment. This will happen because the increased angle of the ramp will increase the initial velocity. Rebound velocity is defined as the velocity of the ball after impacting the side of the pool table. Height is defined as the distance from the top of the ramp to the table at a right angle. The controlled variables include, but are not limited to: ramp used, ball used, length of ramp, length of table, stop watch, meter stick, and ramp and stop watch operators.
Method: To set up the ramp, cover a Tupperware lid with computer paper. Attach two straws parallel to each other so that the ball will follow a set path down the ramp. For each ramp height, measure with the meter stick to ensure that the ramp is at the correct height and with a protractor to make sure that the ramp makes a 90 degree angle with the surface of the table. Make sure that the bottom of the ramp is placed in the same place each time. Use a standard billiard table and standard, American ball (not those damn commy balls!). Place the ramp on the longer side of the table so that the ball will travel a shorter distance and be able to impact the side of the table at each height. Place the ball at the top of the ramp and release it, starting the stopwatch when it impacts the opposite wall. Once the ball has stopped, measure the distance that it traveled with the meter stick. Do five trials for five heights: 2cm, 4cm, 6cm, 8c, and 10cm. Collect data in a table for later analysis.
Diagram:
Materials:
· Billiard ball
· Billiard table
· Tupperware lid
· Computer paper
· Bendy straws
· Meter stick
· Stop watch
· Protractor
Ramp Height, Rebound Distance, Rebound Time Raw Data
|
Height (cm) |
Rebound Distance (cm) |
Rebound Time (s) |
|
2 |
12.2 |
1.7 |
|
2 |
13 |
1.8 |
|
2 |
18.5 |
2 |
|
2 |
17.7 |
2.2 |
|
2 |
12.6 |
1.7 |
|
4 |
55.1 |
4.1 |
|
4 |
40.3 |
3.2 |
|
4 |
54 |
3.6 |
|
4 |
57.7 |
3.7 |
|
4 |
53.5 |
3.5 |
|
6 |
93.4 |
5 |
|
6 |
82.2 |
4.9 |
|
6 |
84.3 |
5 |
|
6 |
85.3 |
4.5 |
|
6 |
77.9 |
4.6 |
|
8 |
101.4 |
5.1 |
|
8 |
105.6 |
5.2 |
|
8 |
104.4 |
5 |
|
8 |
102 |
4.8 |
|
8 |
107.1 |
5.3 |
|
10 |
109.5 |
4.5 |
|
10 |
110.6 |
4.8 |
|
10 |
112.1 |
4.8 |
|
10 |
111.1 |
4.7 |
|
10 |
108.1 |
4.6 |
Height and Calculated Rebound Velocity
|
Height |
Rebound Velocity |
|
2 |
7.176471 |
|
2 |
7.222222 |
|
2 |
9.25 |
|
2 |
8.045455 |
|
2 |
7.411765 |
|
4 |
13.43902 |
|
4 |
12.59375 |
|
4 |
15 |
|
4 |
15.59459 |
|
4 |
15.28571 |
|
6 |
18.68 |
|
6 |
16.77551 |
|
6 |
16.86 |
|
6 |
18.95556 |
|
6 |
16.93478 |
|
8 |
19.88235 |
|
8 |
20.30769 |
|
8 |
20.88 |
|
8 |
21.25 |
|
8 |
20.20755 |
|
10 |
24.33333 |
|
10 |
23.04167 |
|
10 |
23.35417 |
|
10 |
23.6383 |
|
10 |
23.5 |
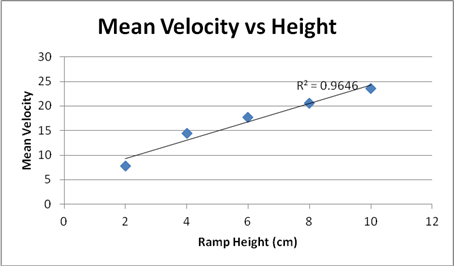
Height and Average Rebound Velocity
|
Height |
Mean Velocity |
|
2 |
7.82 |
|
4 |
14.4 |
|
6 |
17.6 |
|
8 |
20.5 |
|
10 |
23.6 |
Data Files: Text Excel
Graph:
General Form of Calculation: Mean ((X1+X2+X3+X4+X5)/5=mean velocity, where X=velocity for each trial) and velocity calculation (v=s/t where s=distance in cm and t=time in seconds)
Velocity Example:
V=(12.2cm)/(1.7s)
V=7.176471cm/s
Mean Example:
Avg.=( 7.176471+7.222222+9.25+8.045455+7.411765)/5
Avg.=7.82cm/s
Summary: The rebound velocity increases as the height of the ramp increases. The largest increase in velocity took place when the height of the ramp was changed from 2cm to 4cm. The velocity doubled.
Conclusion: The rebound velocity increased as the ramp height increased. With each increase in height, the increase in velocity decreased. For instance, the velocity doubled between 2cm and 4cm, but only increased by about 3cm/s between 8cm and 10cm. Our hypothesis was supported because we predicted that the rebound velocity would increase as the ramp height increased, and it did. This is because of the phenomenon that is expressed in Newton’s Third Law of Motion (see introduction).
Errors: One main source of error was hitting the stopwatch when the ball impacted the wall. It is difficult to be exact when reacting to visual stimuli. The next source of error occurred when measuring the distance traveled by the ball because the sides of the pool table are slanted in .5cm. The next source of error is when measuring the height of the ramp. The height was measured each time, so the actual measurement of the same height could have varied .1cm from the actual height in each trial.
Improvements: To improve this experiment, we could use video analysis to ensure that human error did not alter the time. We could use a different pool table that did not have slanted sides, and we could have used two meter sticks. This would have meant that the one measuring height could have stayed in the same spot the whole time.
Browne, Michael E. (1999-07) (Series: Schaum's Outline Series). Schaum's outline of theory and problems of physics for engineering and science. McGraw-Hill Companies. pp. 58. ISBN 978-0-07-008498-8.
"Equipment". World Snooker Association. London, UK. Retrieved 6 January 2013.
Hibbeler, Russell C. (2009). Engineering Mechanics. Upper Saddle River, New Jersey: Pearson Prentice Hall. pp. 314, 153. ISBN 978-0-13-607791-6.
"Hyatt". Plastiquarian.com. London: Plastics Historical Society. 2002. Retrieved 6 January 2013.
Symon, Keith. Mechanics. Addison-Wesley, Reading, MA. 1971. ISBN 0-201-07392-7.
Shamos, Michael Ian (1993). The Illustrated Encyclopedia of Billiards. New York City: Lyons & Burford. pp. 85, 128 and 168.
William John Macquorn Rankine (1853) "On the general law of the transformation of energy," Proceedings of the Philosophical Society of Glasgow, vol. 3, no. 5, pages 276-280; reprinted in: (1) Philosophical Magazine, series 4, vol. 5, no. 30, pages 106-117 (February 1853); and (2) W. J. Millar, ed., Miscellaneous Scientific Papers: by W. J. Macquorn Rankine, ... (London, England: Charles Griffin and Co., 1881), part II, pages 203-208.
http://www.real-world-physics-problems.com/physics-of-billiards.html This site explained how to solve basic physics problems using billiards.
http://hyperphysics.phy-astr.gsu.edu/hbase/colsta.html This site explained the concepts we used in our research.
http://library.thinkquest.org/TQ0013321/thescience.html This site is about physics theories behind pool.
http://archive.ncsa.illinois.edu/Classes/MATH198/townsend/math.html This site talks about the math we used.
http://www.howstuffworks.com/billiard-table.htm This site explains how pool tables work.