And their use to
Manipulate an Object's Location
By: Erik Brady
Introduction
| Method | Results | Conclusion
| Bibliography | Links | Go Up
Introduction .:. Top
Background
Since the beginning of the
human race, man kind has been aware of sound. As our race progressed, we
began to delve deeper into the mechanics behind sound. It was eventually
discovered that what humans hear in the mind is an electrochemical nervous
impulse that was converted from the mechanical energy of a sound wave
(Assimov). Sound can travel through gas, liquid or even solids in
oscillating patterns called waves. Sound waves rely on a medium, but do
not displace the particles that make up the medium. The wave travels from
particle to particle, and each particle vibrate, knocking into other particles
to transmitting the energy interpreted as sound. Without a medium, sound
waves cannot travel, thus sound cannot travel through a vacuum (Sound Waves).
With variance in mediums and sources of sound, the rate at which sound
travels varies. This rate, known as frequency, is the count of how many
times per second a complete oscillation is made (Pettersson). On a
vibrating medium, nodes and anti-nodes are formed. Nodes are areas where
there is no movement, and contrariwise, anti-nodes are where the medium is
vibrating (Node).
Ernst Florenz Friedrich
Chladni (1756-1827) investigated nodes. By drawing a violin bow across
the edge of a two-dimensional plane, causing vibrations throughout this medium.
By sprinkling sand across the surface, he was able to view the nodal
patterns. The sand would concentrate on the nodes, areas where the
Chladni plate was not vibrating, forming these nodal patterns that came to be
known as Chladni figures. By changing the location of the clamp holding
the plate in place, Chladni achieved patterns. Further experiments with
Chladni plates today project digital frequencies into the plates.
Different frequencies produce different patterns, moving the sand to
different locations (Franceschetti).
Statement of the Problem
The purpose of this
investigation is to determine whether sound waves can be used to visually
depict the nodal patterns in a three-dimensional medium and if by manipulate
frequency, one can change the location of objects in a three-dimensional area.
Hypothesis
I believe that small,
light-weight objects, such as Styrofoam beads, can visually depict the nodal
patterns in a three-dimensional medium, and that by changing the frequency,
thus changing the nodal patterns, the location of these objects can be
manipulated.
Due to current evidence
that nodal patterns can form on a two-dimensional surface when sound waves are
projected into this flat medium, I believe, since often sound travels through
three-dimensional mediums that these nodal patterns are possible in a three
dimensional medium as well. If
this is possible, then by changing the projected frequency, thus changing the
nodal patterns, it should be possible to manipulate the same objects used to
visually depict the nodal pattern.
Variables
The two variables that
will be worked with are the frequency of the sound waves projected into the
medium, and the location of the Styrofoam beads within the medium. The sound frequency is the independent
variable and the location of the beads is the dependent variable.
Other variables that will
be controlled include the tilt of the tube, which will be kept level. The temperature of the medium might
also have an effect on the results.
The dimensions of the tube will also be kept the same throughout the
experiment. These include the
tube’s length, thickness and diameter. Exterior sound will also be kept as silence.
Method .:. Top
Materials
Plastic
tube, one end open (2 in diameter, 73 in long)
◦
To recreate this project, the specifications do not
have to be similar
Large
Speaker (keyboard amplifier)
Styrofoam
cooler (large enough to fit the speaker
A level
Blocks/Books
(Anything to suspend the tube and cooler so that the tube is level)
A digital
tone generator
Styrofoam
beads
Setup
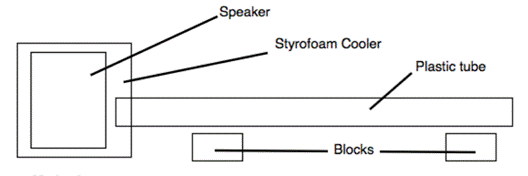

Method

To assemble the setup for
this experiment, place the speaker inside the cooler, then cut a hole into the
side of the cooler parallel with the speaker’s front approximately even with
the center of the speaker. Fill the tube with the desired quantity of
Styrofoam beads, preferably enough that they can be evenly space throughout the
tube in a medium quantity, and gently feed the open end of the tube into this
hole. Using the blocks or books, support the tube and/or cooler and use
the level to determine when the tube is perfectly level.
Plug the speaker into a
power source, and into the digital tone generator. In my case, I used a
laptop connected to the internet, using “The Seventh String Tuning Fork” found
at
www.seventhstring.com.
Try a variety of
frequencies to determine which cause the greatest effects on the Styrofoam
beads. Let each frequency that produces a change in the location of the
beads run for a few minutes until the beads have settled in one location.
Some cause no change, but many cause the beads to form patterns, spaced at
even intervals. Movement in the beads will be observed until they settle
in the node, the point with the least amount of vibration or no vibration.
The observed patterns are the nodal patterns, verifying the first intent
of the hypothesis.
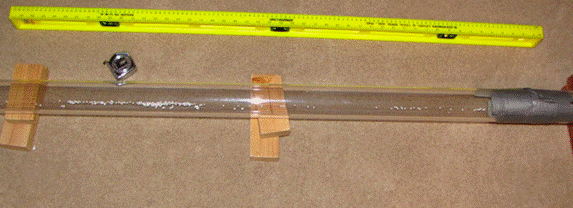
Next, remove all the
Styrofoam beads from the tube, and replace a single bead in the tube.
This solitary bead will react more readily to the sound projected into
the tube than the large group of beads. The goal with this single bead is
to manipulate its movement in a controlled manner. It is easy to use a
variety of frequencies to move the bead from a close point to the sound source
to a farther point.
When trying though, to
return the bead to the starting location, this setup is limited. Although
some frequencies, 659.26 Hz and 164.81 Hz for example, cause the bead to move
towards the sound source, it is never enough to bring it back to the exact
starting location. This verifies in a sense the second purpose of the
hypothesis, controlling an object’s movement with sound. However, we do
not have enough control to completely manipulate the object without more sound
sources.
Results .:. Top

I saw nodal patterns at
multiple frequencies, which supported the first purpose. Most looked like the
pattern pictured at the right.
Though at some frequencies, the clusters were larger.
I was able to move both
large groups of Styrofoam beads and singular beads from one location to the
other, thus supporting the second purpose. It was here that limitations were reached. I could move a large cluster of beads
toward the sound source when projecting a low E (146.83 Hz). But when working with a single bead, I
was unable to move it back towards the sound source more than a few
inches. However, I was able to move
it indefinitely away from the sound source.
To watch the nodal
patterns: physics.m4v
This is a projection of D (146.83 Hz)
and E (164.81 Hz) respectively.
Conclusion .:. Top
Summary and Evaluation of Results
My hypothesis can be
broken down into two purposes. The
first, to visually depict nodal patterns in a three-dimensional medium, can be
partially supported by the nodal patterns I saw at multiple frequencies. However, these patterns remained
two-dimensional at the bottom of the tube. With larger clusters, I a few beads collecting into a second
later at nodal points. Therefore I
believe that with a larger concentration of Styrofoam beads, the visual
depiction of the nodal points will be more three-dimensional.
The second purpose, to
manipulate an object’s location with sound waves, was accomplished in only one
direction. Though a few
frequencies, like an E of 146.83 Hz, displaced the beads in a direction towards
the sound source, it was never enough to exactly control the location of the
beads. Moving away from the sound
source though, I can place the beads in any desired location. I have thus determined that objects can
be manipulated with sound, but the setup to move an object in any desired
direction is far more complex than my own setup, including sound sources in the
opposite direction of any desired direction of movement.
Explanation of Results
The patterns formed due to
the oscillation of waves. This
oscillation forms nodes and anti-nodes.
Nodes are the points of almost no vibration, and anti-nodes are the
points of maximum vibration. The
Styrofoam balls are essentially pushed by the vibrations onto the nodes, thus
forming the nodal patterns depicted.
I was able to move a
single bead away from the sound source because the when the frequency changed,
it would move from the primary nodal point to the new nodal point. The nodal point of the new frequency
that was closer was always farther away from the sound source. It was harder to move the beads closer
to the sound source because the wave was moving away from the source. Some frequencies had more force on the
beads than others. E at multiple
octaves was one of these powerful frequencies. When changing from one frequency to an E, the closest new
nodal points were always closer to the sound source.
Evaluation of Error
One of the largest errors
was static electricity that formed from multiple beads rubbing against each
other constantly. I believe my
most accurate results were when I tested with a solitary bead. When all the possible beads I had
available were poured into the tube and an E was played, they continued moving
towards the sound source without stopping, but a solitary bead would stop at a
nodal point. This can be explained
because the group tried to move towards the nodal point, which was closer to
the sound source. But once there,
there were more beads farther away from the sound source pushing the front of
the cluster towards the sound source.
Thus the whole group continued traveling towards the source, either
pushing each other or pulling due to static electricity.
Another possible error was
in the fact that the end of the tube had many holes covered with duct
tape. This could lead to
irregularities in the sound waves.
Procedure Improvement
To improve the procedure,
I would at least change the set up to include another equal sound source at the
opposite end of a tube with both ends open to more accurately test
movement. I would try to acquire
more Styrofoam beads to test with to see if a tube nearly full of beads will in
fact show three-dimensional nodal patterns. It would also be interesting to see the effects of sound
waves on nodal patterns and the movement of the beads in a gravity-less
environment.
Bibliography .:. Top
Asimov, Isaac.
"Sound." Encyclopedia Americana. Grolier Online, 2010. Web. 26 Oct.
2010.
Franceschetti, Donald R.
"Ernst Chladni's Researches in Acoustics." Science and Its Times. Ed.
Neil Schlager and Josh Lauer. Vol. 4: 1700 to 1799. Detroit: Gale, 2000. 327-329.
Gale Student Resources In Context. Web. 26 Oct. 2010.
"Sound waves."
The Gale Encyclopedia of Science. Ed. K. Lee Lerner and Brenda Wilmoth Lerner.
4th ed. Detroit: Gale, 2008. Gale Student Resources In Context. Web. 26 Oct.
2010.
"Node." Encyclopedia
Americana. Grolier Online, 2010. Web. 26 Oct. 2010.
Pettersson, Peter.
"The Structure and Dynamics of Waves and Vibrations by Hans Jenny."
World-Mysteries.com - Main Menu - SHTML. Web. 27 Oct. 2010. http://www.world-mysteries.com/sci_cymatics.htm.
Links .:.
Top
http://en.wikipedia.org/wiki/Cymatics
This wikipedia page helped
me understand what cymatics is, which got me started in my research.
http://www.world-mysteries.com/sci_cymatics.htm
This is the webpage that
got me most interested in cymatics and prompted me to try using a 3D medium.
This is the online tuning
fork I found particularly useful for my tests.
http://www.youtube.com/watch?v=EprMFajNzfQ
This is the video that
origionally perked my interest in Chladni Plates and the science of sound.
I was prompted to research Chladni
Plates from Mr. Murray.
http://en.wikipedia.org/wiki/Ernst_Chladni
This source helped me learn more
about Chladni himself.
http://www.physics.ucla.edu/demoweb/demomanual/acoustics/effects_of_sound/chladni_plate.html
This is where I further learned
about Chladni Plates.
For a further understanding of
the physics of sound in tubes, I used one of Mr. Murray’s powerpoint
presentations, entitled Standing Waves.
This can be found at:
http://tuhsphysics.ttsd.k12.or.us/DocumentsAndLectures/Lectures/IBI/11-12-SHM-Waves/