Table of Contents | Introduction | Set up | Analysis | Conclusion | Bibliography | Related Links | Return to Research Page |
Background Information: The most common area of basketball to which physics is applied is the act of shooting a basketball. According to Darrick Flores, there are two types of shots: the traditional jump shot with little horizontal velocity and the “running” jump shot with a greater horizontal velocity.[1] Regardless of which type of jump shot is used, putting a backspin on the ball is constant to every shot. The rate of this spin is unique to each individual player, and there is no ideal spin for everyone. Cathy Craig explains that the spin on a ball in flight produces a “Magnus force, which accelerates the ball in a direction that we are unable to process.”[2] Therefore the backspin on a jump shot should have some correlation to the result of the jump shot.
Statement of the Problem: The purpose of this experiment is to identify if an ideal rotational velocity exists when shooting a jump shot.
Review of Literature: Rotational velocity seems to have little effect on the ball as it travels through the air. Curtis Rist explains this when he writes; “The effects of air resistance of the ball are so small because of small velocities, so spin of a shot in [the] air is not useful.”[3] However, backspin must be useful for something, or it would not be taught by shooting coaches world wide. Bill Willis explains further when he says; “The backspin, after contact with the back rim or board, will result in a change in velocity opposite to the spin direction, changing an equal-angle rebound into a velocity more toward the net.”[4] This is because the backspin on the ball would greatly increase the amount of energy lost by the ball due to friction.[5] A ball with little or no backspin would simply bounce off the rim or backboard at the same angle it arrived, away from the net. The backspin of a basketball is also argued to be important as a “teaching element to reproduce the same shot through muscle memory.”[6]
Hypothesis: There will be an ideal rotation rate (in radians per second) on a jump shot from the free throw line that correlates to a greater accuracy on an individual basis. This is because backspin will produce the best possible conditions for a made jump shot according to the literature on the subject.
Set up: The materials we used for the experiment were a high-speed video camera, Logger Pro software to analyze the footage, a basketball, a basketball hoop, and a subject to shoot the “foul line” jump shots. In order to maximize the camera’s view of the basketball rotating, we filmed each jump shot from behind the subject as can be seen in image one.

Our procedure was likewise very simple. One researcher would film the subject with the high-speed camera as the subject took each foul shot. After filming all one hundred foul shots, we downloaded the footage of every jump shot onto the Logger Pro software. Then, using the software we tracked the number of radians the ball rotated and the time elapsed as shown in image one. For each jump shot we measured the rotations in the five clearest, consecutive frames. We also tracked which shot was a make or a miss during the video analysis. Finally, we calculated the rotation rate of each shot by dividing the number of radians the ball rotated by the time elapsed in 5 frames (.45 seconds). There was very little uncertainty for our calculations due to the heavy use of video analysis. However, some uncertainty (.1) still existed in the software used. By using the formula Δx=((Δr/r)+(Δt/t))*x; we determined the average uncertainty to be 2.66.
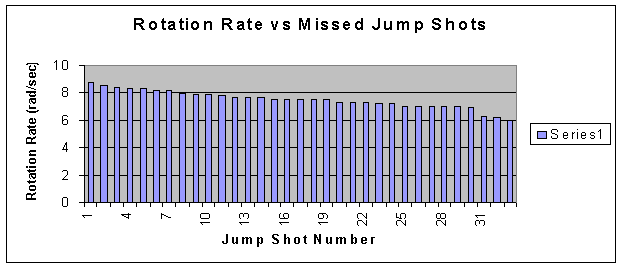
Analysis of the Data: Of the 100 jump shots that were attempted, the subject made 77 of them and missed 33. The average rotation rate of the made foul shots was 6.76 radians per second. This is about a one and one tenth full rotation of the basketball every second. Image two demonstrates the range of the made baskets’ rotation rates is 1.41 radians per second. The graph also demonstrates that the vast majority of the made jump shots had a rotation rate between 6 and 7 radians per second. Only 20 of the 77 made jump shots had a rotation rate outside of that range. This is a strong indication of an ideal rotation rate for a jump shot.
The average rotation rate for
the 33 missed jump shots was also significantly higher than the average
rotation rate of made jump shots at 7.50 radians per second. Image three also
illustrates the significantly higher range of rotation rates for missed jump
shots at 2.78 radians per second.
This range of rotation rates includes values significantly higher than any rotation rate of a made jump shot. For example, the rotation rate of 8.79 radians per second is 1.529 radians per second greater than the highest rotation rate of a made jump shot.
Conclusion: The data supports our hypothesis that there exists an ideal rotation rate on a basketball for greater accuracy on an individual basis. The average rotation rate of a made jump shot was 6.76 radians per second was .74 radians per second lower than the average rotation rate of a missed jump shot of 7.5 radians per second. This indicates that our subject’s ideal rotational velocity is 6.76 radians per second. Made jump shots also demonstrated a greater consistency of rotation rates through its much smaller range. The data also shows that more “backspin” does not necessarily mean improved accuracy as Curtis Rist suggests in his analysis of backspin. Instead, the consistency of the rotation rate of made jump shots suggests the spin of the ball is more beneficial as a teaching aid “teaching element to reproduce the same shot through muscle memory.”[7] The greatest possible source of error comes from the large range of rotational velocities of missed jump shots. This suggests that more than one variable was affecting the missed shots. For example, the subject’s aim could alter slightly from shot to shot. However, the sheer number of data points collected gives our study a good representative sample of our subject’s jump shots. Therefore, we should be able to focus solely on the independent variable we measured (rotational velocity).
Back to Top
Clark, James. www.mrfizzicks.com. October 26, 2007.
Craig, Cathy. Why spinning balls are a curve too far for the human eye. New Scientist, 02624079. Reed Business Information. 3/4/2006. Vol. 189, Issue 2541.
Flores, Darrick A. The Physics of Basketball. 30 April 2003.
Rist, Curtis. The Physics of Foul Shots: Underhanded Achievement. Discover Vol. 21, No 10. October 14, 2003.
Willis, Bill. Physics in Basketball. 2001. 4 October 2003.
-
www.mrfizzicks.com/basketball was great site for acquiring background information.
-
www.worsleyschool.net/science/
files/physicsof/basketball.html is a site providing some interesting information about the role of physics in many aspects of basketball -
http://www.fearofphysics.com/Proj/proj.html provides a fun way to make every jump shot by using physics.
-
www.physics.org is a great website for general physics questions. It is a great place to begin your search for more information.
-
http://www.euclideanspace.com/physics/kinematics/angularvelocity/index.htm provides great information on angular velocities. Although it focuses on particles, it is a great place to begin learning about angular velocity and momentum.