Trebuchets:
The effect of counterweight on the range and velocity of a projectile
Table of Contents:
Background Information:
The trebuchet is an ancient weapon of warfare, first introduced into
siege operations by the French in the twelfth century. It was much more modern
than the catapult or ballista used by the Greeks or Romans. It was the most
effective siege weapon at this time. The force of this weapon differed from the
catapult, as it obtained its projectile force from the terrestrial gravitation
of the counter weight, not from the twisted cordage. From about the middle of
the thirteenth century, the trebuchet was favored mainly due its abilities in
warfare. It had the ability to throw 300 pound stones and more in weight, which
was five or six times more heavy than what the largest catapults of this time
could do. Just one of these stones thrown by the trebuchet could easily break
through the upper parts of the walls of the fortress, which was much more
effective than the need for constant bombardment by the catapults, and allowed
easy access by scaling ladders for the enemy to enter the broken down
fortification. Trebuchets have a long arm with a lightweight sling attached to
the ammunition side and a counterweight much heavier than the ammunition
attached to the other end.
Researching the history of trebuchets is just as important as knowing how
to build, operate, and evaluate the results. Using the trebuchet building kit
makes the assembly of the apparatus easier, as it gives detailed instructions in
a step-by-step method, as to the construction of it. The key to launching the
trebuchet and arriving at the desired results, is to be sure that the launch
angle is kept at a 45-degree angle. In evaluating the results, the use of the
correct formulas is necessary to find the effect of the counterweights on the
velocity and range.
Review of Related Literature-
All
the sites and literature read about in order to prepare for this project ranged
from how-to build your own trebuchet, to students who had done projects on them,
to many gentlemen fascinated with the art of ancient warfare. The webpage by the
Red Stone Project was very useful for the history of trebuchets and contained
pages of detailed information about ancient warfare. Much of our information on
how to adjust the angle for best distances and technique for launching the
trebuchet along with the best types of counterweights came from a packet of
information that came with our trebuchet.
Problem-
The purpose of this investigation is to determine the effect the mass of
the counterweight has on the distance and overall velocity at which the
projectile travels.
Hypothesis-
If the counterweight has a heavy mass in relation to the size of our
trebuchet, then the ball will travel the greatest distance and reach the fastest
velocity, because a light counterweight will not supply enough.
In conducting this experiment we will use the same trebuchet for each
trial, the same setup, and keep the launch angle at 45 degrees.
To ensure that these results will be true for more than one type of
projectile, two balls made of different material will be used. Although the
measurements of the distance and velocity will be different, we predict that the
general affect will be the same. The manipulated variables therefore are the
range and velocity of the projectile after the launch. Distance is defined as
the length the projectile will travel in meters until its first landing point (not
until the ball stops moving). The
overall velocity is defined as the following formula: (vertical velocity^2 +
horizontal velocity^2)^(1/2). The
horizontal velocity is the distance divided by the
time (in seconds) it takes the ball to reach the landing point after the
projectile leaves the trebuchet. The
vertical velocity is 1/2 the gravitational force multiplied by the time.
Equipment-
The equipment used to conduct this experiment mainly consists of the
materials needed to build the trebuchet. The majority of these materials were
provided by a kit which we purchased online at <http://www.trebuchet.com/kit/tabletop/>
. Other materials used for making the trebuchet that were not
in the kit included Elmer's woodcraft glue, a clamp, and a sander.
Additional equipment that was used during the actual experiment was
masking tape, a 25ft tape measure, and a stop watch. The balls we used weigh
0.0269 kg for the iron ball and 0.0068 kg for the wooden ball.
Procedure-
The
first step taken was the construction of our trebuchet.
Using the instructions given to us by those we purchased our kit from, we
were able to complete our trebuchet over the course of several days.
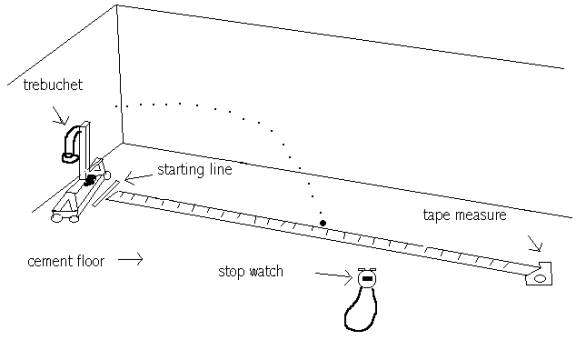
Once we had our trebuchet completed we then set up the space where the
launchings would take place. On a
concrete floor we laid out a tape measure (approximately 25 feet long) in the
direction the trebuchet faced in each of the trials. With masking tape we then marked the starting line at the
beginning of the tape measure. The
trebuchet was then placed with the front of the wheels directly on the tape.
We drew back the arm of the trebuchet and put the pin in the loop to hold
the arm in place. The ball (first the wooden ball) was then set into the pouch.
The pin was pulled out of the loop by a release string to initiate the
launch of the ball, at which time the stop watch began timing.
Time was stopped as soon as the ball hit the ground.
We measured the distance the ball traveled, and recorded both the
distance and time. This procedure
was repeated ten times for each different counterweight. The counterweights included the following: 1.136kg,
1.03kg, 0.91kg, 0.8kg, 0.57kg, 0.45kg, 0.39kg, and 0.31kg. Repeating the same
procedure, including using the same counterweights, the iron ball was then used.
Data Collection-
Preview
Raw Data Here
Calculations-
Formulas Used:
Vertical Velocity-
˝*g*t
Horizontal Velocity- s/t
Overall Velocity- (
(1/2*g*t)^2 + (s/t)^2)^1/2
Average- sum/ quantity
of numbers
Uncertainty- (high
number-low number)/ 2
Conversions Used:
Meters= (2.54* Inches)/100
Kilograms= (lbs/2.2)
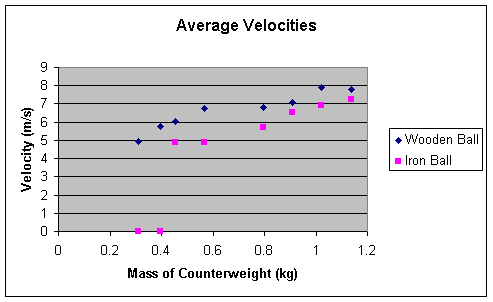
Uncertainty-
Velocity
|
|
Weight
(kg)
|
Average
(m/s)
|
Uncertainty
(m/s)
|
|
Iron
Ball
|
|
|
|
|
|
1.136
|
7.26
|
+/-
.362
|
|
|
1.022
|
6.91
|
+/-
.563
|
|
|
0 .909
|
6.52
|
+/-
.203
|
|
|
0.795
|
5.73
|
+/-
.479
|
|
|
0.568
|
4.86
|
+/-
.798
|
|
|
0.455
|
7.1
|
+/-
.435
|
|
|
0.3125
|
N/A
|
N/A
|
|
|
0.397
|
N/A
|
N/A
|
|
Wooden
Ball
|
|
|
|
|
|
1.136
|
7.79
|
+/-
1.28
|
|
|
1.022
|
7.93
|
+/-
1.48
|
|
|
0.909
|
7.07
|
+/-
1.90
|
|
|
0.795
|
6.79
|
+/-
.953
|
|
|
0.568
|
6.78
|
+/-
1.02
|
|
|
0.455
|
6.04
|
+/-
.988
|
|
|
0.3125
|
4.93
|
+/-
.882
|
|
|
0.397
|
5.78
|
+/-
.983
|
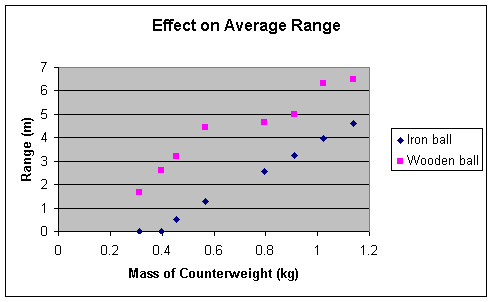
Range
|
|
Weight
(kg)
|
Average
(m)
|
Uncertainty
(m)
|
|
Iron Ball
|
|
|
|
|
|
1.136
|
4.63
|
+/-.229
|
|
|
1.022
|
3.95
|
+/-
.191
|
|
|
0.909
|
3.26
|
+/-
.178
|
|
|
0.795
|
2.57
|
+/-
.152
|
|
|
0.568
|
1.28
|
+/-
.101
|
|
|
0.455
|
.516
|
+/-
.127
|
|
|
0.3125
|
N/A
|
N/A
|
|
|
0.397
|
N/A
|
N/A
|
|
Wooden Ball
|
|
|
|
|
|
1.136
|
6.50
|
+/-
1.76
|
|
|
1.022
|
6.32
|
+/-
2.60
|
|
|
0.909
|
4.98
|
+/-
2.45
|
|
|
0.795
|
4.66
|
+/-
1.28
|
|
|
0.568
|
4.43
|
+/-
.711
|
|
|
0.455
|
3.19
|
+/-
.711
|
|
|
0.3125
|
1.67
|
+/-
.356
|
|
|
0.397
|
2.61
|
+/-
.533
|
Analysis-
In
examining the results which were collected, it is apparent that, in following
the procedure stated, the results were in favor of our hypothesis. For both the
wooden and iron balls, the general affect was consistent in that they went
faster and farther, the heavier the counterweight was. The counterweight of
1.136kg was the heaviest weight used. We
found that this counterweight launched the ball the farthest compared to the
other counterweights. Concentrating on the graphs, the range appears to be a
positive linear function, where as the velocity is a square root function. By
finding the rate, for each ball, we concluded that the approximate rate of
increase was almost equal at 0.177m, which proves the relationship between both
balls is similar. In analyzing the results,
there must be a closer look taken at each ball individually.
The wooden ball proved to be more inconsistent than the iron ball.
Evaluating both the range and the velocity showed that although there was an
overall increase, there was less consistency. This is proved through looking at
the uncertainty of both variables. The higher the uncertainty was, the less
consistent the results.
The iron ball, however, showed more consistency, correlating with the
hypothesis. It showed an overall increase while staying constant. Both the
velocity and the range had lower uncertainties, compared to the wooden ball.
Conclusion-
Our hypothesis was proven correct because the heaviest
counterweight produced
the
farthest distance and the highest velocity in comparison with the other
counterweights
used.
Unfortunately we did not have the supplies necessary to add more weight
to our trebuchet, and we were also skeptical as to whether or not our trebuchet
could even withstand more weight. We suspected that by adding more weight, than
the square root function of the velocity would continue and begin to level out
as it began to reach infinity. To
improve this experiment we would use a broader range of counterweights, and run
more trials for each weight to generate more accurate data. It would also be
beneficial to use sand as a place for the ball to land because finding precisely
where the ball dropped would be easier and more accurate.
Errors and uncertainties could be found in the measurements, such as
using the human eye and memory to locate where the ball dropped, and hand-timing
how long it took the ball to hit the ground.
In addition, my dog picked up the wooden ball in his mouth. We waited for
it to dry, but there still is a possibility that it may have had an effect on
the distance and velocity. If we were to repeat the experiment, the use of a
video camera would have been helpful to track the launch arc of the ball, in
order to have more accurate and more detailed results. We waited for it to dry,
but there still is a possibility that it may have had an effect on the distance
and velocity. A problem that was found early in the experiment involved the
inaccuracy of the launch angle. To fix this problem we changed the formula used
from v=s/t to our current formula which takes into account the angle of the
launch being inaccurate. Another problem found early into the experiment was
that the trebuchet kit only provided two different counterweight possibilities.
In order to be more thorough with our research, we found fishing weights that
had multiple options for use as a counterweight. By using these, we were able to
manipulate the counterweight more effectively.
Works Cited-
“History
and Mechanics of the Trebuchet”
- Kit information and brief historical outline of trebuchets.
www.redstoneprojects.com/trebuchetstore/trebuchet_history.html
Toms,
Ron. “Dedicated to the Art of
Hurling.” October 2002
- A site dedicated to the art of hurling. Contains CD-ROMs, videos and T-shirts.
www.trebuchet.com
Miners,
Russell. “The Grey Company
Trebuchet Page.” February 2000.
Contains
many pictures and information on everything you need to know about trebuchets.
http://members.iinet.net.au/~rmine/gctrebs.html
Fuzzball
Software. “Trebuchet Home Page
and Trebuchet FAQ.” 1998
- Explores Frequently Asked Questions about trebuchets.
http://www.belfry.com/fuzzball/trebuchet/
Radlinski,
Filip. “Welcome to the Physics of
the Trebuchet.” 1997.
- Kind of like our website.
http://www.geocities.com/SiliconValley/Park/6461/trebuch.html
www.victoriassecret.com-



