Pre-Quiz 3.2 .:. Go Up
Projectile Motion
Show your work, circle your answers, and use significant digits to receive full credit.
1-3: A ball rolls off the edge of a cliff. The instant it leaves the edge, it has an initial horizontal velocity of 17.3 m/s, and it strikes the ground after 1.56 seconds.
|
1. How high is the cliff? (11.9 m)
2. How far from the base of the cliff does the ball land? (27.0 m)
3. What is the speed of impact? (23.1 m/s) |
Horizontal s u = 17.3 m/s v a = 0 (horizontally) t = 1.56 s |
Vertical s u = 0 (purely horizontal) v a = -9.81 m/s/s (on earth) t = 1.56 s |
Solution:
So in the vertical direction you can use v = u + at (Not in the packet - you must memorize it!!!) to find the final vertical velocity:
v = 0 + (-9.81)(1.56) = -15.3036 m/s
And you can find the vertical displacement using s = ut + 1/2at2:
s = 0 + 1/2(9.81)(1.56)2 = 11.936 m (This is the answer to 1)
In the horizontal direction you can find the displacement using s = ut + 1/2at2: - (a = 0), so this becomes s = ut
s = ut = (17.3)(1.56) = 26.988 m (Answer to 2)
As for the speed of impact, the velocity of impact is 17.3 m/s in the x direction, and -15.3036 m/s in the y, the speed is the hypotenuse of this:
= sqrt(17.32 + 15.30362) = 23.097 m/s (The answer to 3)
Questions 1, 2 and 3 are to see if you can solve a cliff problem:
4-5: A ball is launched at 43.2 m/s at an angle of 25.2o above horizontal on a level field.
|
4. How far does the ball go before striking the ground? (147 m)
|
Horizontal s = u = 39.089 m/s (see below) v = 39.089 m/s a = 0 (horizontally) t = 3.7500 s |
Vertical s = 0 u = 18.394 m/s v = -18.394 m/s a = -9.81 m/s/s (on earth) t = 3.7500 s |
Solution:
If you get an angle magnitude vector to start with, you have to break it into components. If the 25.2o is with the x-axis, then it is the trig angle, and the initial x and y components are:
Vx = 43.2cos(25.2o) = 39.089 m/s
Vy = 43.2sin(25.2o) = 18.394 m/s
We know in the vertical direction two other things, s = 0 (The field is level), and that v = -18.394 m/s (it hits the ground going down as fast as it was going up to begin with)
It is a simple matter to use v = u + at (if you remember it) to find time in the vertical direction:
-18.394 = 18.394 + (-9.81 m/s/s)t
t = 3.7500 s
And finally to find the horizontal displacement:
s = ut + 1/2at2
a = 0, so
s = ut = (39.089 m/s)(3.7500 s)
s = 146.581 = 147 m
|
5. There is a very tall wall 130. m away. With what velocity does the ball strike the wall? (Express your answer as an angle-magnitude vector – draw a picture) (41.6 m/s @ 20.0o below horizontal)
|
Horizontal s = 130. m u = 39.089 m/s (see below) v = 39.089 m/s a = 0 (horizontally) t = 3.3258 s
|
Vertical s = ? u = 18.394 m/s v = ? a = -9.81 m/s/s (on earth) t = 3.3258 s
|
Solution:
If you get an angle magnitude vector to start with, you have to break it into components. If the 25.2o is with the x-axis, then it is the trig angle, and the initial x and y components are:
Vx = 43.2cos(25.2o) = 39.089 m/s
Vy = 43.2sin(25.2o) = 18.394 m/s
We are also told that the wall is 130 m away, so this is the horizontal displacement s.
We can solve the horizontal for the time:
s = ut + 1/2at2
a = 0, so
s = ut , 130. m = (39.089 m/s)t
t = 3.3258 s
Now we find the final vertical velocity using v = u + at
v = 18.394 m/s + (-9.81 m/s/s)(3.3258 s)
v = -14.232 m/s (it is already going down)
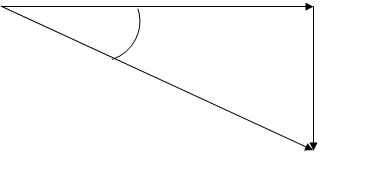
If we draw this vector, it looks like this
Where the horizontal is 39.089 m/s, and the vertical is -14.232 m/s. The magnitude (speed) is the hypotenuse = sqrt((39.089 m/s)2 + (-14.232 m/s)2) = 41.6 m/s, (Be careful of squaring negative numbers with your TI graphing calculator)
and the angle indicated is tan-1(14.232/39.089) = 20.0o (below the horizontal) .:.
Questions 4 and 5 are to see if you can solve an arc trajectory problem, and solve for obscure stuff somewhere in the middle of the trajectory