Problem Set 6.10: | 35
| 37 |
40 | 41 | 58 | 42
|
43 | 63 | 64 | 44
| 46 | 67 | 69 | 75
| Go up
- by Cooper Spear and Chris Murray, 2002
35. Jane, looking at Tarzan, is running at top speed (5.6 m/s) and grabs a vine hanging vertically from a tall tree in the jungle. How high can she swing upward? Does the length of the vine (or rope) affect your answer?
Tarzan wasn't a ladies man he'd just come along and scoop 'em up under his arm like that, quick as a cat in the jungle.
Jane, on the other hand, is a real lady, so let's set this up.
This is a classic Conservation of energy problem:
Total Energy Before
= Total Energy After
Before:
Jane running along the level ground with a velocity of 5.6 m/s. She has kinetic energy, but nothing else.= After:
Jane having swung up to some height h, and momentarily at rest. Here she has potential energy, and nothing else.1/2mv2
=
mgDh
m cancels from both sides, giving
1/2mv2 = mgDh
1/2v2 = gDh
( 1/2v2 )/g = Dh
1/2(5.6 m/s)2/(9.8 m/s/s) = 1.6 m
Assuming the vine is longer than 1.6 m, the length does not matter, the longer the vine, the smaller the angle she swings through to reach a particular height.
(Table of contents)
37. A sled is initially given a shove up a frictionless 25.0 ° incline. It reaches a maximum vertical height 1.35 m higher than where it started. What was its initial speed?
Total Energy Before
= Total Energy After
Before:
The sled moving along the ground before it has gained any height. It has kinetic energy and nothing else= After:
The sled having slid up the plane and come to a rest It has potential energy and nothing else.1/2mv2
=
mgDh
1/2mv2
=
m(9.8 m/s/s)(1.35 m)
m cancels from both sides giving:
1/2mv2 = mgDh
1/2v2 = gDh
v2 = Ö{2gDh} = {2(9.80 N/kg)(1.35 m)}
v = 5.14 m/s
Thankfully, the degree of the incline is not relevant.
(Table of contents)
40. A roller coaster, shown in Fig. 6-38, is pulled up to point A where it and its screaming occupants are released from rest. Assuming no friction, calculate the speed at point B, C, D. C's height is 25 m, D's height is 12 m.
In this problem the potential energy at point A is turned into some combination of potential and kinetic at the other points. Arbitrarily, and conveniently, let's decide that the potential energy at Point B represents Zero Potential energy, as it is the lowest point.
Point B:
Total Energy Before
= Total Energy After
Before:
The rollercoaster is at point A, up at the top of the hill at rest. It has Potential energy, and nothing else.= After:
The Rollercoaster is at the bottom of the hill. It has kinetic energy, but no potential, as we have decided that the elevation at B is Zero.mgDh
=
1/2mv2
Now, we don't know the mass, but it is in every term, so let's just cancel it:
mgDh = 1/2mv2
gDh = 1/2v2
2gDh = v2
v = Ö{2gDh} = Ö{2(9.8 m/s/s)(30 m)} = 24.25 m/s = 24 m/s
Point C:
Total Energy Before
= Total Energy After
Before:
The rollercoaster is at point A, up at the top of the hill at rest. It has Potential energy, and nothing else.= After:
The Rollercoaster is at Point C. It has both kinetic energy and potential energy. (It is at height 25 m)mghA
=
1/2mv2 + mghC
Now, we don't know the mass, but it is in every term, so let's just cancel it:
mghA = 1/2mv2 + mghC
ghA = 1/2v2 + ghC
ghA - ghC = 1/2v2
2(ghA - ghC) = v2
Ö{2g(hA - hC)} = v
Ö{2(9.80 m/s/s)(30 m - 25 m)} = v
v = 9.9 m/s
Point D
Total Energy Before
= Total Energy After
Before:
The rollercoaster is at point A, up at the top of the hill at rest. It has Potential energy, and nothing else.= After:
The Rollercoaster is at Point D. It has both kinetic energy and potential energy. (It is at height 12 m)mghA
=
1/2mv2 + mghD
Now, we don't know the mass, but it is in every term, so let's just cancel it:
mghA = 1/2mv2 + mghD
ghA = 1/2v2 + ghD
ghA - ghD = 1/2v2
2(ghA - ghD) = v2
Ö{2g(hA - hD)} = v
Ö{2(9.80 m/s/s)(30 m - 12 m)} = v
v =18.8 m/s = 19 m/s
(Table of contents)
41. A projectile is fired at an upward angle of 45.0 ° from the top of a 265-m cliff with a speed of 185 m/s. What will be its speed when it strikes the ground below? (Use conservation of energy)
Since we just want to know the speed, (and not the angle) we don't need to solve the projectile motion stuff we can look at it upon its launch, and at the moment of impact with the ground.
Total Energy Before
= Total Energy After
Before:
The projectile is at the top of the 265 m tall cliff, and moving at 185 m/s. It has both potential and kinetic.= After:
The projectile is just striking the ground. It has no potential, only kinetic1/2mv12 + mgh
=
1/2mv22
Now, we don't know the mass, but it is in every term, so let's just cancel it:
1/2mv12 + mgh = 1/2mv22
1/2v12 + gh = 1/2v22
v12 + 2gh = v22
Ö{v12 + 2gh} = v2
Ö{(185 m/s)2 + 2(9.8 m/s/s)(265 m)} = 198.54 = 199 m/s
(Table of contents)
58. How long will it take a 1750-W motor to lift a 285-kg piano to a sixth-story window 16.0 m above?
Assuming the lifting is done at a constant velocity. (i.e. the change in potential energy is the entire amount of energy transformed) then we can use the formula for Power:
P = W/t - Where W is not just work as in W = Fs, but any transformation of energy, so
W in this case = DEp = mgDh = (285 J)(9.8 N/kg)(16.0 m) = 44688 J
Now if P = W/t, then t = W/P = (44688 J)/(1750 W) = 25.536 s = 25.5 s
(Table of contents)
42. A 60-kg bungee jumper jumps from a bridge. She is tied to a 12-m-long bungee cord and falls a total of 31 m. (a) Calculate the spring constant k of the bungee cord. (b) Calculate the maximum acceleration experienced by the jumper.
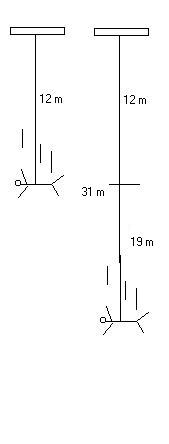
When they reach the end of the Bungee cord, and begin to stretch it, they have fallen 12 meters, and then they stretch it an additional distance of 19 m. (31 - 12 = 19) When they come to a halt, we know that the total change in potential energy (Due to the change in height equal to 31 m) has been stored in the spring.
Total Energy Before
= Total Energy After
Before:
The jumper is on the bridge and has potential energy and nothing else= After:
The Bungee jumper has come to rest stretching the cord distance s, and having fallen a total distance (s + 12m) They have elastic potential and nothing else.mgh
=
1/2ks2
So let's solve for k:
mgh = 1/2ks2
2mgh/s2 = k = 2(60 kg)(9.80 m/s/s)(31 m)/(19 m)2 = 100.99 N/m = 1.0x102 N/m
Now we had better solve for the maximum upward acceleration of the jumper. It occurs at the maximum stretch of the bungee cord, as that is when the stretch distance is the greatest. The formula for the force exerted by a spring is:
F = -kx = -(100.99 N/m)(-19 m) = 1918.74 N upwards
Now our expression of Newton's second law has the weight downward (-) of (60 kg)(9.80 N/kg) = 588 N, and the force exerted by the bungee cord of 1918.74 N upwards (+):
<1918.74 N - 588 N> = (60 kg)a
a = 22.2 m/s/s = 22 m/s/s upwards
(Table of contents)
43. A vertical spring (ignore its mass), whose spring constant is 900 N/m, is attached to a table and is compressed 0.150 m. (a) What speed can it give to a 0.300-kg ball when released? (b) How high above its original position (spring compressed) will the ball fly?
This has two parts, a) is from the lowest position, to the ball just leaving the spring. (which occurs .150 m above the initial position)
Total Energy Before
= Total Energy After
Before:
The ball is on the spring, at rest and at the lowest position. It has elastic potential energy, but nothing else.= After:
The ball is just leaving the spring which is fully extended. It has potential and kinetic energy and nothing else.1/2ks2
=
mgh + 1/2mv2
So let's solve for velocity:
1/2ks2 = mgh + 1/2mv2
1/2(900 N/m)(.150 m)2 = (.300 kg)(9.80 m/s/s)(.150 m) + 1/2(.300 kg)v2
v = 8.035 m/s = 8.03 m/s
Part b) is from the lowest position to the ball up in the air, having risen some height h into the air.
Total Energy Before
= Total Energy After
Before:
The ball is on the spring, at rest and at the lowest position. It has elastic potential energy, but nothing else.= After:
The ball at its highest point, having risen some distance "h" into the air. It has gravitational potential and nothing else.1/2ks2
=
mgh
So let's solve for height:
1/2ks2 = mgh
(1/2ks2)/mg = h
{1/2(900 N/m)(.150 m)2}/(.300 kg)(9.80 m/s/s) = 3.44 m
(Table of contents)
63. How much work can a 3.0-hp motor do in 1.0 h?
3.0 hp = (3.0 hp)(745.7 W/hp) = 2237.1 W
1.0 h = 3600 s
P = W/t, so W = Pt = (2237.1 W)(3600 s) = 8053560 = 8.1 x 106 J
(Table of contents)
64. A shot-putter accelerates a 7.3-kg shot from rest to 14 m/s. If this motion takes 2.0 s, what average power was developed?
The transformation of energy in this case is a change in kinetic energy:
P = W/t = (1/2mv2 )/t = (1/2(7.3 kg)(14 m/s)2 )/(2.0 s) = 357.7 W = 360 W
(Table of contents)
44. A small mass m slides without friction along the looped apparatus shown in Fig. 6-39. If the object is to remain on the track, even at the top of the circle (whose radius is r), from what minimum height h must it be released?
We know that at the top of the loop, the velocity must be such that the centripetal acceleration is equal to "g", so
g = v2/r, so v2 = gr. Since the formula for kinetic energy uses v2, I will not take the square root.
We also know that at the top of the ramp, it has potential energy, and at the top of the loop, it has both potential and kinetic:
Total Energy Before
= Total Energy After
Before:
The mass is at the top of the ramp at rest. It has gravitational potential energy, but nothing else.= After:
The mass is at the top of the loop. It has both kinetic and potential energy, but nothing else.mgh
=
1/2mv2 + mgh2
At the top of the loop, it is at a height of 2r, where r is the radius of the loop:
mgh = 1/2mv2 + mgh2
mgh = 1/2mv2 + mg2r
now v2 = gr, so
mgh = 1/2mgr + mg2r
Divide by mg:
h = 1/2r + 2r = 2.5r
(Table of contents)
46. An engineer is designing a spring to be placed at the bottom of an elevator shaft. If the elevator cable should happen to break at a height of h above the top of the spring, calculate the value that the spring constant k should have so that passengers undergo an acceleration of no more than 5.0 g when brought to rest. Let M be the total mass of the elevator and passengers.
If you want the maximum upward acceleration to be 5.0 "g"s, then the maximum upward force the spring exerts on the elevator would need to be 6 times the weight of the elevator. (As 1 times the weight of the elevator upward results in no upward acceleration). If you prefer to be non voodoo, let's use Newton's second law: We have upward F the force of the spring, and Mg the weight of the elevator. so:
<F - Mg> = M(5g), and F = 6Mg.
Now if this upward force is from a spring compressed some distance s, the maximum upward acceleration comes just as the spring is compressed to its maximum distance. then F = ks = 6Mg, so s = 6Mg/k
But what is the compression of the spring? well, using energy, the elevator starts at the top some distance h from the spring, and undergoes a total change in height equal to h + s, so we have gravitational potential energy turning into elastic potential of the spring:
Mg(h+s) = 1/2ks2
And plugging in our expression for s:
Mg(h+6Mg/k) = 1/2k(6Mg/k)2
Mgh + 6M2g2/k = 1/2k(36M2g2/k2)
Mgh + 6M2g2/k = 1/2k(36M2g2/k2)
gh + 6Mg2/k = 18Mg2/k
gh = 12Mg2/k
h = 12Mg/k
k = 12Mg/h
(Table of contents)
67. How fast must a cyclist climb a 6.0 ° hill to maintain a power output of 0.25 hp? Neglect work done by friction and assume the mass of cyclist plus bicycle is 70 kg.
P = (.25 hp)(745.7 W/hp) = 186.425 W
P = W/t = Fs/t, since W = Fs
Now, s/t = v, so P = Fs/t = Fv, where v is the velocity.
As the bicyclist climbs the hill, if we neglect friction, which is a ridiculous notion, the only force opposing them is the component of gravity parallel to the hill = mgsin(q) So we have
P = Fv = mgsin(q)v, and
v = P/mgsin(q) = ( 186.425 W)/{(70 kg)(9.8 N/kg)sin(6.0o)} = 2.6 m/s
(Table of contents)
69. Squaw Valley ski area in California claims that its lifts can move 47,000 people per hour. If the average lift carries people about 200 m (vertically) higher, estimate the maximum total power needed.
First off, they need to change the name of the ski area. Squaw is not a polite reference to a woman. It is a slang reference to female genitalia. (a good translation into English slang starts with "c" and rhymes with "runt") I found this out in a most embarrassing way when I was a teacher at an Indian school.
The power needed to just lift the people, if we assume that they have a mass of about 70 kg (154 lbs on Earth)
Then P = W/t, and in this case, W = mgh, so
P = W/t = mgh/t = (47,000 people)(70 kg/person)(9.8 N/kg)(200 m)/(3600 s) = 1791222.222 W = 2 MW
(Table of contents)
75. A .20 kg pinecone falls from a branch 18 m above the ground. (a) With what speed would it hit the ground if air resistance could be neglected? (b) If it actually hits the ground with a speed of 10.0 m/s, what was the average force of air friction exerted on it?
(a) This is simple, potential (mgh) turns all to kinetic (1/2mv2)
mgh = 1/2mv2
v = Ö(2gh) = Ö(2gh) =18.78 m/s = 19 m/s
(b) This is a little more subtle.
At an elevation of 18 m, the pinecone has mgh = (.20 kg)(9.8 N/kg)(18 m) = 35.28 J of energy
But, it arrives at the ground having only 1/2mv2 = 1/2(.20 kg)(10.0 m/s)2 = 10. J of kinetic energy, which means it lost 35.28 J - 10. J = 25.28 J of energy to friction on the way down due to work against friction:
W = Fs
25.28 J = F(18 m), F = 1.4 N. The book makes it negative as I suppose. Hmm, why would they do that. Because the work was negative? (i.e. the air took energy away from the pinecone?) It would have been an upward force on the pinecone anyway...CJM
(Table of contents)