Problem Set 3.8: | 35 | 36 | 38 | 41 | 43 | 45 | 49 | 51 | 58 | 63 | 69 | 71 | 73 | Go up
 - by To Chong, Chris Murray, Sonja Scherer, and Cooper Spear,  2001

35. A projectile is fired with an initial speed of 75.2 m/s at an angle of 34.5° above the horizontal on a long flat firing range. Determine (a) the maximum height reached by the projectile, (b) the total time in the air, (c) the total horizontal distance covered (that is, the range), and (d) the velocity of the projectile 1.50 s after firing. 

Solve for b and c first:

Horizontal	Vertical
s = ? u = 75.2cos34.5o = 61.9743 m/s v = " a = 0 t = ?	s = 0 u = 75.2sin34.5o = 42.5937 m/s v = -42.5937 m/s (hitting level ground) a = -9.8 m/s/s t = ?
	Use: v = u + at -42.5937 = 42.5937 + -9.80t t = 8.6926 s = 8.69 s (b)
Use: vav = Ds/Dt 61.9743 m/s = Ds/8.6926 s s = 538.7 m = 539 m (c)

now solve a:

Horizontal	Vertical
s = ? u = 75.2cos34.5o = 61.9743 m/s v = " a = 0 t = ?	s = ???? u = 75.2sin34.5o = 42.5937 m/s v = 0 (at the top) a = -9.8 m/s/s t = ?
	Use: v2 = u2 + 2as 02 = (42.5937)2 + 2(-9.8 m/s/s)s s = 92.56 m = 92.6 m (a)

now solve d:

Horizontal	Vertical
s = ? u = 75.2cos34.5o = 61.9743 m/s v = " a = 0 t = 1.50 s (given)	s = ? u = 75.2sin34.5o = 42.5937 m/s v = ? a = -9.8 m/s/s t = 1.50 s (given)
	Use: v = u + at v = 42.5937 m/s + -9.8 m/s/s(1.50 s) = 27.8937 m/s

 so the velocity at 1.50 seconds is 
61.9743 m/s x + 27.8937 m/s y (in vector component notation)
It has a magnitude of (61.9743² + 27.8937²)^1/2 = 67.96 = 68.0 m/s
At an angle of tan^-1(27.8937/61.9743) = 24.2^o above the horizontal (d)

(Table of contents)

36. A projectile is shot from the edge of a cliff 125 m above ground level with an initial speed of 105 m/s at an angle of 37.0°  with the horizontal as shown in Fig. 3-39. (a) Determine the time taken by the projectile to hit point P at ground level. (b) Determine the range X of the projectile as measured from the base of the cliff. As the instant just before the projectile hits point P, find (c) the horizontal and the vertical components of its velocity, (d) the magnitude of the velocity , and (e) the angle made by the velocity vector with the horizontal.

Horizontal	Vertical
s = ? u = 105cos37 = 83.8567 m/s v = " a = 0 t = ?	s = -125 m u = 105sin37 = 63.1906 m/s v = ? a = -9.8 m/s/s t = ?
	Use: v2 = u2 + 2as v2 = (63.1906 m/s)2 + 2(-9.8 m/s/s)(-125 m) |v| = 80.2686 m/s but v must be going down so v = -80.2686 m/s (part of c)
	Use: v = u + at -80.2686 m/s = 63.1906 m/s + (-9.8 m/s/s)t t = 14.6387 s = 14.6 s (a)
Use: vav = Ds/Dt 83.8567 m/s = Ds/14.6387 s s = 1227.5528 m = 1230 m or 1.23 km (b)

Part (c):
when it hits the ground the velocity components are:
83.8567 m/s x + -80.2686 m/s y or 83.9 m/s x + -80.3 m/s y (c)

Part (d):
This has a magnitude of
(83.8567² + 80.2686²)^1/2 = 116.08 m/s = 116 m/s (d)

Part (e):
Which forms an angle of
tan^-1(80.2686/83.8567) = 43.7^o below the horizontal (e)

(Table of contents)

38. A rescue plane wants to drop supplies to isolated mountain climbers on a rocky ridge 325 m below. If the plane is traveling horizontally with a speed of 250 km/h (69.4 m/s), (a) how far in advance of the recipients (horizontal distance) must the goods be dropped (Fig. 3-41 a) ? (b) Suppose, instead that the plane releases the supplies a horizontal distance of 425 m in advance of the mountain climbers. What vertical velocity (up or down) should the supplies be given so that they arrive precisely at the climbers' position (Fig. 3-41 b) ? (c) With what speed do the supplies land in the latter case?  

Part a:

Horizontal	Vertical
s = ???? u = 69.4 m/s v = " a = 0 t = ?	s = -235 m u = 0 v = ? a = -9.8 m/s/s t = ?
	Use: s = ut + 1/2at2 -235 = 0 + 1/2(-9.8 m/s/s)t2 s = 6.9253 s
Use: vav = Ds/Dt 69.4 m/s = Ds/6.9253 s s = 480.61 m = 481 m (a)

Part b and c:

Horizontal	Vertical
s = 425 m u = 69.4 m/s v = " a = 0 t = ?	s = -235 m u = ???? (b) v = ? a = -9.8 m/s/s t = ?
Use: vav = Ds/Dt 69.4 m/s = 425 m/Dt t = 6.1239 s
	Use: s = ut + 1/2at2 -235 m = u(6.1239 s) + 1/2(-9.8 m/s/s)(6.1239 s)2 u = -8.367 m/s = -8.37 m/s (down) (b) (the solution manual is wrong - they use a rounded time (6.12 s) and they shouldn't have)
	Use: v = u + at v = -8.367 m/s + (-9.8 m/s/s)(6.1239 s) v = -68.3813 m/s

(69.4 m/s² + 68.3813 m/s m/s²)^1/2 = 97.429 m/s = 97.4 m/s (c)
(Table of contents)

41. Huck Finn walks at a speed of 1.0 m/s across his raft (that is, he walks perpendicular too the raft's motion relative to the shore). The raft is traveling down the Mississippi River at a speed of 2.7 m/s relative to the river bank (Fig. 3-43). What is the velocity (speed and direction) of Huck relative to the river bank?

 

Across	Downstream
s = ? v = 1.0 m/s t = ?	s = ? v = 2.7 m/s t = ?

The magnitude is:
(1.0 m/s² + 2.7 m/s²)^1/2= 2.897 m/s = 2.9 m/s (magnitude)
The direction is:
tan^-1(2.7 m/s/1.0 m/s) = 70.^o downstream of straight across (or 20.^o from the shore)
(Table of contents)

43. A boat can travel 2.30 m/s in still water. (a) If the boat points its prow directly  across a stream whose current is 1.20 m/s, what is the velocity (magnitude and direction) of the boat relative to the shore? (b) What will be the position of the boat, related to its point of origin, after 3.00 s? (See Fig. 3-30.) 

Part a: 
The magnitude is:
(2.30 m/s² + 1.20 m/s²)^1/2 = 2.594 m/s = 2.59 m/s (magnitude)
The direction is:
tan^-1(1.20 m/s/2.30 m/s) = 27.553 =  27.6^o downstream of straight across (or 62.4^o from the shore)

Part b:

Across	Downstream
s = ? v = 2.30 m/s t = 3.00 s	s = ? v = 1.20 m/s t = 3.00 s
Use: vav = Ds/Dt 2.30 m/s = Ds/3.00 s s = 6.90 m	Use: vav = Ds/Dt 1.20 m/s = Ds/3.00 s s = 3.60 m

So the boat is 6.90 m across and 3.60 m downstream
The magnitude (distance in this case) is:
(6.90 m² + 3.60 m²)^1/2 = 7.783 m = 7.78 m (distance)
The direction is:
tan^-1(3.60 m/6.90 m) = 27.553 =  27.6.^o downstream of straight across (or 62.4^o from the shore)

Or you could use the velocity from part a: 2.594 m/s 62.4^o from the bank
s = vt = 2.594 m/s(3.00s) = 7.78 m 62.4^o from the bank
(Table of contents)

45. An airplane is heading due south at a speed of 500. km/h. If a wind begins blowing from the southwest at a speed of 100. km/h (average), calculate: (a) the velocity (magnitude and direction) of the plane relative to the ground, and (b) how far off course it will be after 10 min if the pilot takes no corrective action. (Hint: First draw a diagram.)

v_wind = 70.71 km/hr east + -70.71 km/hr south

East	South
s = ? v = 70.71 km/hr t = 10/60 hr = .166666 hr	s = ? v = 500 - 70.71 = 429.28 km/hr t = 10/60 hr = .166666 hr
Use: vav = Ds/Dt 70.71  = Ds/.166666 hr s = 11.78 km  = 10 km off course (b)

The magnitude is:
(70.71 km/hr² + 429.28 km/hr²)^1/2 = 435.1 km/hr = 435 km/hr (magnitude)
The direction is:
tan^-1(429.28 km/hr/70.71 km/hr) = 80.64^o =  80.6^o south of east (or 9.36^o east of south)
(Table of contents)

49. A motorboat whose speed in still water is 3.60 m/s must aim upstream at an angle of 27.5º (with respect to a line perpendicular to the shore) in order to travel directly across the stream. (a) What is the speed of the current? (b) What is the resultant speed of the boat with respect to the shore? (See Fig. 3-28.)

 

Across	Downstream
s = ? v = 3.60cos27.5 = 3.1932 m/s = 3.19 = velocity of boat (b) t = ?	We know that there is no net velocity downstream because the upstream component of the boast's velocity exactly cancels out the current: vb = 3.60sin27.5 = 1.662 m/s s = ? v = vc - 1.662 m/s = 0 so current = 1.66 m/s (a) t = ?

(Table of contents)

51.A swimmer is capable of swimming 1.00 m/s in still water. (a) If she aims her body directly across a 150-m-wide river whose current is 0.80 m/s, how far downstream (from a point opposite her starting point) will she land? (b) How long will it take her to reach the other side?

Part a and b:

Across	Downstream
s = 150 m v = 1.0 m/s t = ?	s = ? v = .80 m/s t = ?
Use: vav = Ds/Dt 1.0 m/s = 150 m/s/Dt t = 150 s (b)
	vav = Ds/Dt .80 m/s = Ds/150 s s = 120 m (a)

(Table of contents)

58. William Tell must split the apple atop his son's head from a distance of 27 m. When he aims directly at the apple, the arrow is horizontal. At what angle must he aim it to hit the apple if the arrow travels at a speed of 35 m/s?

Use range equation

q = sin^-1(g(range)/v²)/2

The necessary angle is:

q = sin^-1(g(range)/v²)/2 = 6.24degree
The angle 90 - 6.24 = 83.76degree is unreasonable.  (it would also strike his son)

The full story of William Tell is interesting.  He was forced to shoot the apple off his own son's head because his legendary marksmanship and headstrong manner were threatening to a pompous lord.  After successfully shooting the apple from his son's head, another arrow fell from his cloak.  The lord asked what the second arrow was for, Tell replied "The second arrow was for you had my son come to harm"

Don't mess with headstrong bowmen.

(Table of contents)

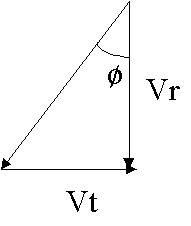
63. Raindrops make an angle q with the vertical when viewed through a moving train window (Fig. 3-48). If the speed of the train is  v_t , what is the speed of the raindrops v_d in the reference frame of the Earth in which they are assumed to fall vertically?

  tanq = Vt/Vr ==> Vr = Vt/tanq

Vt is the speed of the trin

Vr is the speed of the rain

(Table of contents)

69. The cliff divers of Acapulco push off horizontally from rock platforms about 35 m above the water, but they must clear rocky outcrops at a water level that extend out of the water 5.0 m from the base of the cliff directly under their launch point. See Fig. 3-49. What minimum pushoff speed is necessary to do this? How long are they in the air?

Horizontal	Vertical
s = 5.0 m u = ? v = " a = 0 t = ?	s = -35 m u = 0 v = ? a = -9.8 m/s/s t = ?
	Use: s = ut + 1/2at2 -35 m = 0 + 1/2(-9.8)t2 t = 2.6726 s =  2.7 s (b)
The necessary speed to do this is: vav = Ds/Dt vav = 5.0 m/2.6726 s = 1.8708 m/s = 1.9 m/s (a)

(Table of contents)

71. Agent Tim, flying a constant 185 km/h horizontally in a low-flying helicopter, wants to drop a small explosive onto a master criminal's automobile traveling 145 km/h on a level highway 88.0 m below. At what angle (with the horizontal) should the car be in his sights when the bomb is released (Fig. 3-51)?

Horizontal	Vertical
s = ? u = 185 - 145 = 40 km/hr/3.6 = 11.11111 m/s v = " a = 0 t = ?	s = -88.0 m u = ? v = ? a = -9.8 m/s/s t = ?
We have the initial velocity of the bomb is: 11.1111 m/s	Use: s = ut + 1/2at2 -88.0 m = 0 + 1/2(-9.8)t2 t = 4.2378 s
The distance from the base it will land is: vav = Ds/Dt 11.1111 m/s = Ds/4.2378 s s = 47.0870 m

The angle is tan^-1(s_vertical/s_horizontal) = tan^-1(88/47.0870) = 61.8 degrees below horizontal.
(Table of contents)

73. A projectile is launched from ground level to the top of a cliff which is 195 m away and 155 m high (see fig 3-52).  If the projectile lands on top of the cliff 7.6 s after it is fired, find the initial velocity of the projectile (magnitude and direction).  Neglect air resistance.

Horizontal	Vertical
s = 195 m u = ? v = " a = 0 t = 7.6 s	s = +155 m u = ? v = ? a = -9.8 m/s/s t = 7.6 s
vav = Ds/Dt vav = Ds/Dt v,u = 25.6579 m/s	s = ut + 1/2at2 s = ut + 1/2at2 u = 57.6347 m/s

The magnitude is:
( 25.6579 m/s² + 57.6347 m/s²)^1/2 = 63.0880 m/s = 63 m/s (magnitude)
The angle is tan^-1(57.6347/25.6579) = 66.0023^o = 66^o degrees above horizontal.
(Table of contents)