AP Problems Chapter 1-4: | 1
| 2 | 3 | 4 | Go
up
- by Aaron Pride, 2003
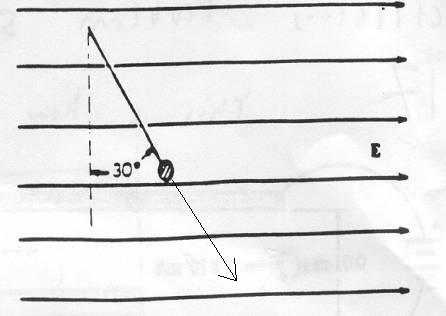
1. A small conducting sphere of mass 5 x 10-3 kilogram, attached to a string of length 2 x 10-1 meter, is at rest in a uniform electric field E, directed horizontally to the right (see diagram). There is a charge of 5 x 10-6 coulomb on the sphere. The string makes an angle of 30º with the vertical. Assume g = 10 m/s2 (sin 30º = ½ cos 30º = Ö3/2 tan 30º = Ö 3/3)
Diagram:
In the space below, draw and label all forces acting on the sphere.
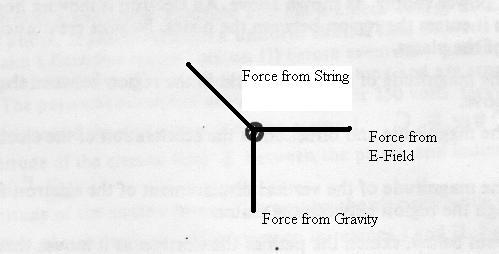
Calculate the tension in the string and the magnitude of the electric field.
First we take the angle of the string (30 degrees from the vertical), and the cosine of that because we are looking for the hypotenuse. Next we take the adjacent force (remember SOH CAH TOA) which is the force of gravity acting on the sphere. So our equation is cos 30 = ((5e-3)*9.8)/x where x is the force of tension on the string. The tension on the string is .058 N. The second part of the problem, where we calculate the magnitude of the electric field, can be solved by taking the force generated by the electric field and plugging it into the E = F/q formula. Here we are using tan, because we need to find the adjacent force.
So, tan(30) = x / (5e-2) the force acting on the sphere by the E field is .0288 N, and when we plug that into the formula, we get E = (.0288) / (5e-6), so our magnitude is 5774 N/C, or 5.8e3 N/C
The string now breaks. Describe the subsequent motion of the sphere and sketch on the following diagram the path of the sphere while in the electric field.
The sphere would move in the same direction it is pointing, the direction that the string is being pulled towards.
2. A pair of square parallel conducting plates, having sides of length .05 meter, are .01 meter apart and are connected to a 200-volt power supply, As shown below. An electron is moving horizontally with a speed of 3e7 m/s when it enters the region between the plates. Neglect gravitation and the distortion of the electric field around the edges of the plates.
Diagram:

a) Determine the magnitude of the electric field in the region between the plates and indicate its direction on the figure above.
The electric field can be found using the E = V/d formula. We take the voltage over the distance between the plates and we get the E field. So, E = 200/.01, so our E field magnitude is 2e4 V/m.
b) Determine the magnitude and direction of the acceleration of the electron in the region between the plates.
We take the formula Eq=ma (as they both equal force) and do some simple algebra and we can get the acceleration. So our formula is
a = (2e4 * 1.602e-19)/ (9.11e-31) = 3.517 E 15 m/s/s our acceleration is 3.5e15 m/s2.
c) Determine the magnitude and vertical displacement of the electron for the time interval during which it moves through the region between the plates.
| Horizontal | Vertical |
| s = .05 m
u = 3 E 7 m/s v = 3 E 7 m/s a = 0 t = .05/3E7 = 1.66666 E-9 s
|
s
u = 0 v a = 3.517 E 15 m/s/s t
|
Here we will use SUVAT. First we
will find the time, by using the T = S/V, so our time is T = .05/ 3e7, our
time equals 1.666e-9. From there we can use the ut + .5at2 = S to
find the vertical displacement. Our equation is (0 * 1.666e-9) + (.5 *
3.5e15 * 1.666e-92) = .004885 m,
S= 4.9e-3 m
d) Sketch the path of the electron as it moves through and after it emerges from the region between the plates.
It would be parabolic, concave upward in the region between the plates - barely avoiding the upper plate (by a tenth of a cm), and pretty straight (assuming we can neglect the 9.8 m/s/s of gravity) once it left the plate region

3. An electron initially moves in a horizontal direction and has a kinetic energy of 2e3 eV when it is in the position shown below. It passes through a uniform electric field between two oppositely charged horizontal plates (region 1) and a field-free region (region 2) before eventually striking a screen at a distance of .08 meter from the edge of the plates. The plates are .04 meter long and are separated from each other by a distance of .02 meter. The potential difference across the plates is 250 volts. Gravity is negligible.
Diagram:
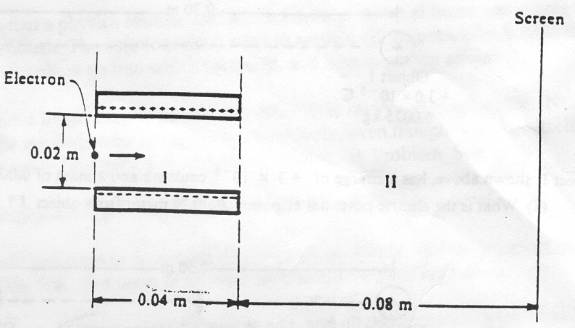
a) Calculate the initial speed of the electron as it enters region
1. First we take and make kinetic energy equal to the voltage times charge, so
Vq = ½ mv2 or
(2E3 V)(1.602e-19C) = ½ * (9.11e-31 kg)*v2
so v2 = (2E3 V)(1.602e-19)/ ((1/2)*9.11e-31)
or v = 2.652E7 = 2.7e7 m/s
b) Calculate the magnitude of the electric field E between the plates, and indicate its direction on the diagram above.
E = V/d, or E = 250/.02 E=12500 V/m
c) Calculate the magnitude of the electric force acting on the electron while it is in region 1.
E = F/q, or F= 12500 * 1.602e-19 F=2.0e-15 N
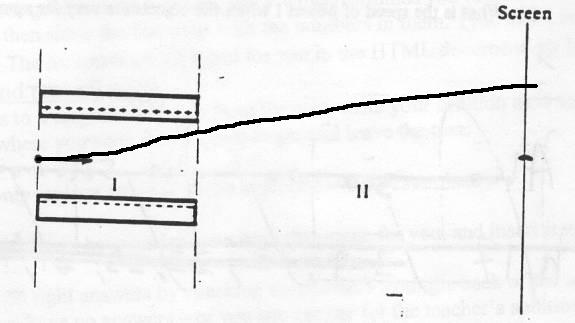
d) On the diagram below, sketch the path of the electron in regions 1 and 2. For each region, describe the shape of the path.
Diagram: (parabolic, concave upward in region I, nearly straight in region II)
4. Object 1, as shown below, has a charge of 3e-6 coulomb and a mass of .0025 kg.
Diagram:

a) What is the electric potential at point P, .3 meter from Object 1?
V = kq/r, or V= k *3e-6 / .3 V = 89900 Volts
b) Object 2, of the same mass as Object 1, but having a charge of 1e-6 coulomb, is brought from infinity to point P, as shown below.
Diagram:

c) How much work must be done to bring the object 2 from infinity to point P?
V = kq1 / r, or V=89900 V… W= Vq2, or W= 89900 * 1e-6 W= .0899 J
d) What is the magnitude of the electric force between the two objects when they are .3 meter apart?
E = kq1q2 / r2, or E = (k*3e-6 * 1e-6) / (.3)2 F = .29966 = .30 N
e) What are the magnitude and direction of the electric field at the point midway between the two objects?
So we are .15 from each charge, object 1 creates an electric field to the right on the point in the middle equal to:
E = kq/r2, or E = k * 3e-6 / .152 = 1.1987 E 6 N/C to the right
Object 1 (the 1E-6 C charge) creates an electric field to the left at the center equal to:
E = kq/r2, or E = k * 1e-6 / .152 = 3.9956 E 5 N/C to the left
So the net electric field at the central point is the vector sum of these
1.1987 E 6 N/C to the right - 3.9956 E 5 N/C to the left = 7.99 E 5 N/C to the right
E= 8e5 N/C to the right