(Final Conclusion)

Finding the Eddy Current Force
TOContents
Previous____Next

Contents
Graph 2.2
Graph 2.3

Graph 2.2 TOC

Data File

From my second experiment I believe it can be concluded that the relationship in between the eddy current torque and angular velocity is a linear relationship, as is evident in Graph 2.2. I then proceeded to confirm this by doing lines of best fit. The results were of course that a line made the best fit. The coefficients of correlation for these lines were from 0.9954 at 5.08 mm to 0.9998 at 2.54 mm. I next tried to check this data with my data from the first section. If the magnetic field, as my hypothesis suggested, were linear in relation to the eddy force, the difference in magnetic field strength would manifest itself in the differences in slope. So I made a graph of the slopes of the six lines versus the distance of the magnet. The slope, since Angular Velocity vs. Torque proved linear, will represent the coefficient between distance and B-field.

Graph 2.3 TOC

Data File

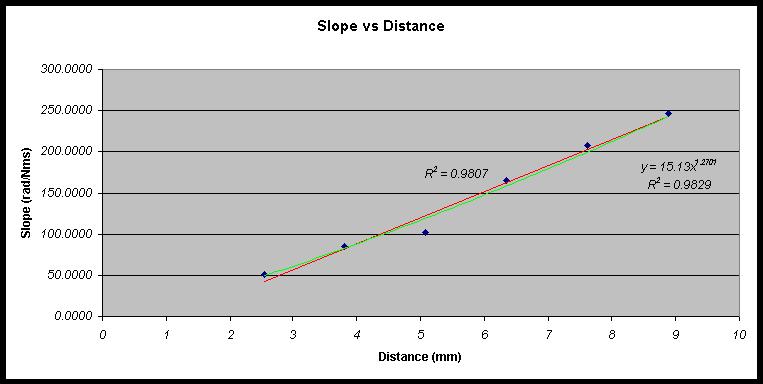
If the magnetic field had directly affected the eddy currents as of a linear relationship it sort of could be explained by the graph. The graph had concluded a relationship of B to r of about r^1.278 (green line, Graph 2.3), which would comply only sort of with the idea that a magnet’s power proportionality decreases over the difference if we take into account the results from the first experiment. Since the power to which r is taken according to the first experiment decreases as we get closer, it is possible that it decreased to the point of 1.278, but not certain. I would very much like to reconcile this problem, and maybe find the formula for decrease in power to which r is taken over the distance for a permanent magnet. Another problem is that the graph of slope versus distance can also be fairly accurately fit by a linear equation (red line, Graph 2.3). This would suggest not that the relationship between magnetic field strength and magnetic eddy current is linear but that it is some negative root proportionality such as r -(1/3), or r –(1/2). Another explanation is that maybe a more dispersed magnetic field could cause a higher eddy current than one less dispersed. I based this on the fact that it is harder to create a movement of current to a place where charge separation already is. In the eddy currents case this would mean that with magnets closer it would be a little harder to produce eddy currents and with magnets farther, a little easier. However, this doesn’t make too much sense considering the speed of electricity. This entire problem, however, may just be attributed to human error. There were many places for human error to be involved. For example, when timing the weight’s speed error came into play. This margin of error was about ± 0.2 rad/s in each of the final results. Also, there was uncertainty of ± 0.0035 Nm about my answer for frictional torque. I also had many problems trying to get the magnets at just the right distances. This is seen in the Graph 2.3 by the distance of 5.06 mm, which from the line of best fit seems to suggest the magnet was actually at a separation of 4.5 mm. I noticed, furthermore, that the plate wobbled a bit and was not precisely centered. 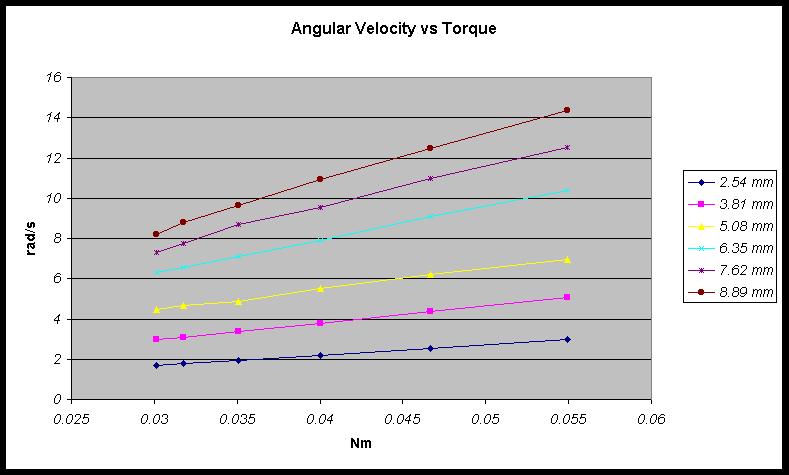Overall, I was only really certain that the relationship between eddy current torque and angular velocity was a linear relationship and a little less convinced of the linear relationship between magnetic field and eddy current torque. Also, I was very much swayed about the relationship in between magnetic field and distance being linear. I now see this relationship as a regressing power function, contrary to my hypothesis. A useful test to conclude other answers would be a better method to measure the B-field versus distance, maybe one using a Hall-effect probe. Another useful experiment would be one where I both spread out small, eddy producing magnets, and then compacted them to see if dispersion really is a factor.

Previous____Next