
Research Report by Matt Hadfield

TABLE OF CONTENTS
INTRODUCTION | HYPOTHESIS | METHOD | PROCEDURE |
CALCULATIONS | CONCLUSIONS | VARIABLES| LINKS | Research Page
INTRODUCTION | TABLE OF CONTENTS
The most popular winter sport in the
world is skiing. The sport of skiing
requires certain specialized equipment.
One needs skis, as well as; poles for balance and rhythm, and two hard
shelled boots for stability. Skiing, as
a professional sport, requires one other major piece of equipment; wax. Wax is applied to the bases of the skis to
reduce the amount of friction between the skis and the snow. In general, the less friction the faster the
speed. I have skied all my life and
have just come to know, in the last few years, that wax is very important. Proper application and type of wax can
severely improve one’s performance. As a ski racer, I have been immersed into
the world of wax and have not yet come to understand it. The wax I refer to, is not the wax of a
candle, but a combination of chemicals and substances combined to increase
speed. There are several different
types of ski wax. For instance, some
waxes are theoretically for extreme cold days and others for warmer days. Supposedly, if I was to use a cold day wax
made for application and use in temperatures of 10-30 degrees Celsius during a
day with temperatures around 32-35 degrees Celsius, then my speed would be
considerably lower than its potential.
This could result in a race time two or three seconds from the leader
thus dropping me to the bottom of the standings! For these reasons, it is said to be very important to use the
correct wax. For a beginner, this
probably sounds like a foreign language, but for me, it’s my native
tongue. I want to know what the best
wax is so I can perform at an optimum level.
I will test the four different
standard waxes. I will use the colors
yellow, red, blue, and green of Toko brand wax. Each of these has a supposed temperature range for optimum
performance. The yellow wax is
theoretically for temperatures of zero to negative four degrees
Fahrenheit. Red for temperatures of
negative four to negative ten degrees Fahrenheit. Blue is for temperatures of negative ten and lower. Finally green is supposedly and all
temperature wax for the entire spectrum of temperatures. I hope to discover the properties of these
waxes. Does wax choice make a
significant difference in performance?
HYPOTHESIS | TABLE OF CONTENTS
I believe that I will find that
there is a very significant difference in performance between the waxes. I believe I will find that the waxes perform
in a logical order. The wax supposed to
be applied at the calculated temperature should win. In the case of the all temperature wax I believe it will finish
second. This is because its properties should make the wax adapt to the
temperature it is emerged in, but I think it will fail to out perform the wax
designated for the temperature.
To test my hypothesis, I want to
design an experiment that will have the fewest variables that could possibly
alter my results. I am trying to test
four different types of wax that should all have different velocities when
tested. I will create a uniform,
measured track that I can time the different waxes on. I want a slope that is less than ten degrees
so that there will be less gravitational force pulling the skis down the hill
than on a slope of greater degree. The
skis that I will use for the experiment are very important. I will take two identical skis and cut them
in half in the exact middle. This will
give me four even ski sections. I will
take the four different waxes and apply one of them to each base. Now to make my experiment more realistic, I
will add some sort of weight to each ski so that there is a mass to ski
ratio. This is important because humans
obviously weigh down on the skis. I
will secure this mass to each ski section.
Now I will need a suitable track to complete this experiment. I will allot an area of a slope of S meters
and create my track. The track is to be
approximately two inches deep and three inches wide. By digging in the track, I will have a more uniform base for
experimentation. Next I will have to
record the temperature of the snow. The
temperature of the snow is what one is supposed to use when determining what
wax to use on any given day. This makes
the temperature very important in this experiment. Next, I will test my hypothesis by running each ski section down
the track and recording its time. I will
repeat this process of running the skis down the track until I have enough data
and it seems fairly consistent with each sections previous trial times. This will make the results more conclusive
by having a broader base of values for each wax type. I figure that I will time each section about five times. That will give me a total of twenty data
points from which to draw my conclusions.
With the calculated times, I can then calculate the velocity at which
each ski section travels as well as their accelerations. From these calculations I will be able to
clearly see which wax was the fastest and draw conclusions relating to my
hypothesis.
On the test day, the conditions were
perfect. The sky was virtually clear
and the sun was out. This was a big
relief considering that the weather conditions are a large variable. I will explain the importance of the weather
condition later in this report.
I chose an area that had a nice
uniform slope. I chose to have a fairly
small angular slope for various reasons that I will also explain later in this
report. I measure off a track length
that should allow the skis to attain their maximum velocity. The track I constructed was approximately
648 inches long. This value converted
to meters equals 16.4592, or about 16.5 meters. After marking off the track length, I, with the help of my fellow
physicist Lindsay Wilson, began constructing the track. The area I chose was ungroomed, which means
not packed snow, so I first ran a full ski down the area to give myself a rough
outline as to where I would cut the track in.
After creating the outline, I scraped away all excess snow laying on top
of the packed snow base leaving a smooth surface to begin digging. Next, I began shoveling out the track. I did this with a flat faced shovel by
sticking the blade approximately five centimeters into the track outline and
then scooping out the snow. After
crudely hollowing out the track, I went back to the top and began packing and
smoothing the track length. I did this
with a full ski by running it continuously up and down the track and firmly
pressing the ski into the track base. I
then made sure that the surface of the track base was smooth and void of all
major bumps and small crevices that may alter the path of the test
subjects. As I was working to construct
this track, I realized that it would be almost impossible to make this track
perfectly straight, level, and uniform, and had to settle for the best possible
track I could create. I ended up with a
almost level and straight track that should work nicely for my experiment. Diagrams of the track are below.
SKI TRACK
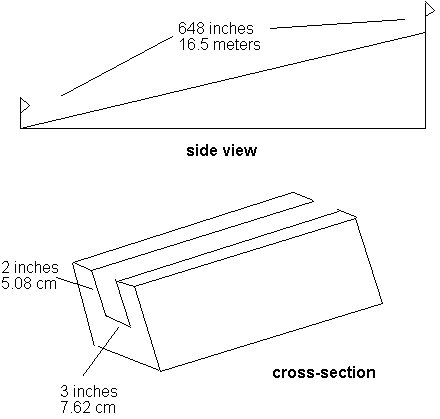
Now that the track was constructed,
I poured water dyed with red food coloring across the start a finish lines to
make them more visible. This would cut
back on the possibility of human error on the timing aspect of this
experiment. Next, I began to calculate
the angle of the slope. The slope of
the hill will effect the velocity of the skis as I will explain later in this
report. I did this using two
methods. One involving a protractor
with a hanging weight and the other using a level and a ski.
The protractor method follows the
laws of geometry. By hanging a weight
from zero degrees down to the plane of angles, one can calculate the angle of
any slope. I used sewing thread and a
small fishing weight in the construction of this device. I used a small thread so that the weight of
it will not effect the measure of the degree.
I mounted my protractor arc side down on a ruler. I made the flat edge of the protractor and
the flat edges of the ruler all parallel to each other. Now by laying the edge of the ruler onto the
snow, the weight will theoretically stay aligned with gravity, straight down,
and I can measure the angle on the protractor.
This theory, explained using a diagram and geometry is below.
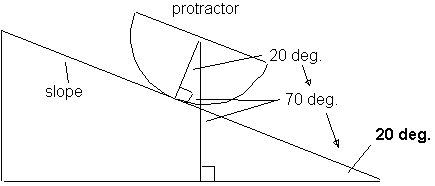
I took six readings of the angle of
the slope of the track at various points along it. The values in degrees, in descending order, are as follows: 25,
18, 9, 7, 6, 6. Now by taking the
average, I will should have the average angle of the slope.
25 + 18 + 9 + 7 + 6 + 6 = 71 ------>
71/ 6 = 11.8 degrees.
The
average angle of the slope, calculated using this method was 11.8 degrees.
The other method of calculating the
angle of the slope involves using a level and a tall object. I set the level flat on the snow at the
starting line of the track, so that the bubbles in the level read parallel to
the ground. Next, I stuck my whole ski
into the snow straight up at the point of the finish line. By looking down the flat edge of the level,
I could then mark off the line where my vision leaves the level and hits the
straight ski. I did this and determine
the height to be approximately six feet, or 1.83 meters. By using geometry, I can now use that
measured height, along with the measured length of the track, to determine the
angle of depression. This is done by
following the laws governing triangles.
A diagram of the procedure and the subsequent calculations is below.
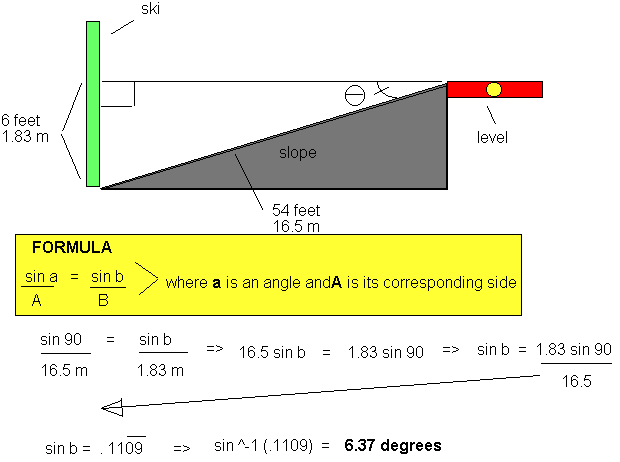
The angle of the slope using this
method, was 6.4 degrees. Now comparing
the two values, there is a large discrepancy.
The value calculated using the protractor, is almost twice a large as
the value calculated using the level.
This was an unforeseen problem. I thought that, theoretically, the two
values would be almost identical. What
I now know, in the terms of this experiment, is that the method using the level
is more accurate. I realized that I did
not uniformly and evenly take the measures of the slope with the
protractor. I had taken the first two
measurement close to the top of the track where the slope is steeper than at
the bottom. I should have taken more measurements and spaced them out
evenly. This would have made my
calculation of the angle much more precise.
For this reason, the other method is much more credible. Theoretically, the protractor method would
be more accurate because the measurements at the given point is exact; where as
in the experiment with the level, the measurement is an approximation due to
the distance from the level to the ski, and the falsities of the human
eye. Therefore, I will use the slope
angle of 6.4 degrees for this experiment.
This fits into my desired method which was to have a slope angle of less
than ten degrees.
As I explained in the method section
of this report, the temperature of the snow is theoretically a determining
factor as to what wax should be applied on any given day. I brought two thermometers to the test site
to record the temperature of not only the snow, but also the air. I decided to record the temperature of the
air so that I would be able to observe my eventual results compared to both the
temperatures to see if there was similarities or differences in them. When I was setting up and testing my
experiment, I placed the outdoor thermometer out of direct sunlight and not
touching the snow so that the temperature would not be altered by these outside
factors. I brought a medical
thermometer to place in the snow. I stuck
this into the snow near the track. When
I had calculated the angle of the slope, I then went to check and record the
temperatures. The air temperature at
the site was 28 degrees Fahrenheit or -2.2 degrees Celsius. Next I went to check the temperature of the
snow. I realized as I was looking for
the level of the mercury, that this thermometer started degree readings at 94
degrees Fahrenheit. I did not know this
beforehand and had to compensate for this mistake. I then stuck the outdoor thermometer into the ground. After the experiment, I went and recorded
the temperature of the snow. The
temperature of the snow was 25 degrees Fahrenheit or -4 degrees Celsius. This data will be extremely important when
drawing a conclusion after the experiment.
The next step was the actual
experimentation. The ski sections were
to be ran down the track and their time recorded. For this experiment, I had my assistant stand at the bottom of
the track to start and stop the stopwatch and record the times. I was to sit at the top and prepare the skis
for each trial and yell out when to start the watch. I chose to run all four sections consecutively instead of running
one section continuously for x number of trails. This, I thought, would make the experiment more precise by
exposing the sections to virtually the same track condition continuously. I ran them in the following order: blue wax, yellow wax, all temperature wax,
red wax. This order was selected at
random but I continued to run the experiment in this order. As I stated in the method section, I was
going to continue running trials until I had enough values and they seemed
fairly consistent. I ended up running
seven trials for each section. I
decided to stop at seven because the values for each section were all fairly
close and consistent. The values of
each section, in terms of the time they took to run the track each trial, is
below.
RED
YELLOW BLUE ALL TEMPERATURE
Trial 1 7.2 5.94 6.7 5.94
Trial 2 6.9 5.34 5.91 5.39
Trial 3 5.99 5.56 5.81 6.07
Trial 4 6.38 5.78 5.96 6
Trial 5 5.83 5.58 5.63 5.63
Trial 6 5.29 5.4 5.65 5.58
Trial 7 6.01 5.64 5.44 5.72
Values in seconds
DATA
FILE
Now from these values I can first
calculate the average time, then I can calculate the average velocity, and
finally I can determine the average acceleration of the ski sections. From all this data, I can prove or disprove
my hypothesis and make several connections and conclusions to my original
research question.
CALCULATIONS | TABLE OF CONTENTS
Now that I have taken the necessary
data and performed my experiment, the next step is to compute the data and
analyze the calculations. With hand
timing there is an unavoidable uncertainty.
I will explain this variable later in this report. I have elected to drop the highest and
lowest time values from each ski section.
This will give a closer range of values for each wax thus creating a
more precise calculated average time value.
For this variable of human error I have chosen an uncertainty value of
plus or minus two-tenths of a second.
The time averages with their uncertainties are shown below.
RED YELLOW
7.20
5.94
6.90 Average
5.78 Average
6.38 31.11 / 5
5.64 27.96 / 5
6.01 6.222 sec + - .2
5.58
5.592 sec + - .2
5.99 6.02 - 6.42
5.56 5.39 - 5.79
5.83
5.40
5.29
5.34
BLUE ALL TEMPERATURE
6.70
6.07
5.96 Average
6.00 Average
5.91 28.96 / 5
5.94 28.87 / 5
5.81 5.792 sec + - .2
5.72 5.774 sec + - .2
5.65 5.59 - 5.99
5.63 5.57 - 5.97
5.63 5.58
5.44 5.39 Values in seconds
DATA FILE
From these values, I can now calculate the
average velocities at which the skis traveled down the track. The formula for this is V average = change S / change T where S is the track distance and T
is the time. The track distance, which
is 16.5 meters, is a constant in this equation. I will calculate the average velocities of the highest, lowest,
and average value of T for all waxes.
The calculations are below.
RED velocity
YELLOW
velocity
High t 6.42 2.57 5.79 2.85
Average t
6.22 2.65
5.59 2.95
Low t 6.02 2.74 5.39 3.06
BLUE velocity
ALL TEMP velocity
High t 5.99 2.76 5.97 2.76
Average t
5.79 2.85
5.77 2.86
Low t 5.59 2.95 5.57 2.96
t in seconds
v in meters per second
DATA FILE
With these velocities, I can now
calculate the accelerations of the four different waxes. The formula for acceleration is A average =
change V / change T. I will plug in the
three calculated values of velocity from above for V and also the corresponding
values of T from above. The values of change V are the same as V average because the ski starts from
rest and reaches that velocity so the change is equivalent to V average. The calculations for acceleration are bellow.
RED YELLOW
velocity/time ACCELERATION velocity/time ACCELERATION
2.57/ 6.42 = 0.4 2.85/ 5.79
= 0.49
2.65/ 6.22 = 0.43 2.95/ 5.59
= 0.53
2.74/ 6.02 = 0.46 3.06/ 5.39
= 0.57
BLUE ALL
TEMP
velocity/time ACCELERATION velocity/time ACCELERATION
2.76/ 5.99 = 0.46 2.76/ 5.97
= 0.46
2.85/ 5.79 = 0.49 2.86/ 5.77
= 0.5
2.95/ 5.59 = 0.53 2.96/ 5.57
= 0.53
acceleration in meters per
second squared (m/s^2)
DATA FILE
CONCLUSIONS | TABLE OF CONTENTS
In my hypothesis, I stated that I
hoped to find that there is a significant difference between the waxes
performance and that there should be a logical order between them. From analyzation of the calculations I have
run into a problem. The logical order
should be that yellow “wins” followed by red, and blue. The all temperature wax I believed would
fall in between yellow and red, or second place. It did finish second, but in front of blue. These results are apparent when analyzing
average velocities. The quirk lies in
the results of blue. Blue ousted
red. Theoretically, blue wax is
designated for temperatures of negative ten degrees Fahrenheit and lower and
red wax was created for temperatures of negative four to negative ten
degrees. The temperature of the snow on
the day of experimentation was approximately negative four degrees
Celsius. This fall directly into the
theoretical path of red wax. It should
have performed much better than blue.
The reason for this error is not possible for me to pin. The best possible idea I came up with was
that the ski sections which I cut were physically different. I thought that maybe the original two front
sections of the skis possibly performed better. I examined my ski sections and found that blue wax section was
not a front section but a tail section of the original ski disproving that
theory. No other solutions for this
quirk are valid. My experiment is valid
and uniform making this result awkward and contradictory to my thesis.
The waxes did yield different
results. In this 16.5 meter frame of
reference the results do seem very close.
In the terms of an actual ski race, the results would much more spaced
apart. Consider an actual race course where
the times would be 25 times the experimented values. To do this I will just multiply the average time for each wax
color by 25.
Red = 6.22 x 25 => 155.5 seconds
Yellow = 5.59 x 25 => 139.75 seconds
Blue = 5.79 x 25 => 144.75 seconds
All
Temp = 5.77 x 25 => 144.25 seconds
Now the differences seem much more
obvious. For example the difference
between yellow and blue is five seconds.
This is a very large difference in terms of ski racing. That five seconds would separate the winner
and the loser in a good ski race. Also
as I stated in my hypothesis, I believed the all temperature wax would be
second but would not win because it is not formulated precisely for any given
temperature like the set colored waxes are.
The accelerations also follow the
route of the velocities. This meaning
that yellow, having the fastest velocity, also has the quickest
acceleration.
In the method section of this
report, I stated that I wanted to create an experiment with as few variable as
possible. In the design and creation of
this experiment, I found several things that are variables and I had to make a
scientific decision as to what route to take dealing with them. The first variable I came across was whether
to select a slope of large degree or a slope of small degree. I deduced that on a slope of large degree,
the force of gravity is greater and acts on the ski by pulling it down the
slope at a greater velocity. With the
added force of gravity, the ski wax is acting less on its own. The results would possibly show less
difference in velocity between the ski sections because gravity is outweighing
the different waxes. On a shallower
slope, gravity is pulling less on the ski to travel down the hill. Of course, gravity is the reason the ski
glides in the first place, but with less slope angle, the benefits of wax are
more obvious. For these reasons, I
chose a slope of less than ten degrees for the experiment.
Another variable in this experiment,
was how to create the track. My initial
idea was to have no set track, just an area to start the ski and a finish line
to stop the timing. There are positive
aspects to this approach. This format
would be more natural and realistic to the actual conditions within which a ski
performs. Also with no dug-in track,
there is no possibility that the ski will press against the walls of the track,
thus causing friction and slowing the ski down. In a track format, the ski is subject to a uniform length on
which to travel. This in turn causes a
uniform data that is collected. With no
track, the skis would perform in various different ways. For example, the red waxed ski might travel
a path more to the right than the yellow waxed ski which travels at a
straighter path to the finish line.
This in turn would cause the distance traveled (S) of the red ski to be
greater than the distance traveled by the yellow ski and thus would foul up the
eventual calculations of the velocity.
By choosing a set track format, I have eliminated this variable and made
my experiment more credible.
The weather conditions are a very
important variable. In the event of bad
weather, which would consist of any of the following; snow, rain, or major
wind, would alter the experiment. If,
for example, it was snowing on my day of experimentation, the continuous
addition of snow to my track would cause the track to change its physical shape
and uniformity. The alteration in this
case would make my results of the testing to be spangled and chaotic thus
making it hard to draw conclusions due to lack of cohesive data. The weather on the day of my
experimentation, as I mentioned earlier, was optimal for testing. This weather variable I overcame due mostly
to luck and the weather channel.
The actual ski which the wax was
applied to is a variable. I had a hard
time deciding whether or not to cut the ski into segments or to leave it
whole. Both options have
advantages. If the ski was to remain
whole, the testing would be closer to the realistic event of skiing. With whole skis there would be no
possibility of significant physical differences between the two test
subjects. The two skis would be
identical and the only difference between them would be the wax applied to the
base. Unfortunately, with this method I
would only be able to test two types of waxes.
I thought about waxing two different pairs of skis with different waxes
but then realized that the physical difference between the two pairs would be
significant enough to greatly alter the results of the experiment. For example, if I was to wax a pair of old
skis, which I did, and a pair of good skis, the better pair of skis should
result in faster times in the track because of their technological advantage
and their better physical shape they are in.
I also thought about testing the old pair with tow different waxes on
their bases, then stripping and melting the wax off of them and adding the two
new waxes to their bases and testing them.
This would be the ideal route to doing this experiment but due to time
constraints this is not possible. A
good wax job allows the wax to cool for several hours before use on snow. If I was to test the first waxes then
re-wax, I would not be able to start testing again for several hours, within
which the weather conditions would change due to the position of the sun along
with the temperature of the air and snow.
By cutting the skis into two sections each of equal form, I was able to
do all the testing of all four waxes in one sitting at the same general
time. This makes the test more valid
because there is a more uniform testing site that is less affected by time.
The actual timing of the ski
sections is another variable. When
using a stopwatch, there is the obvious event of human error. The mind and the body do not work in perfect
unison, so when your brain says “start the watch” it takes x percent of a
second to respond. Also the timing is
uncertain because I, as the starter, could have yelled out “go” x percent of
second too early or too late from the actual release of the ski. The optimal way to time each ski section
would be to use laser timing equipment like that of an actual ski race. This equipment was unavailable to me for
this experiment. Fortunately, human
error is actually very small. I have
trust in my assistant that she has good reflexes which she claimed. For this reason I earlier stated an
estimated uncertainty due to human error of two-tenths of a second plus or
minus the calculated value. This does
have an effect on the results but the effect is not conclusion altering.
Official web site of TOKO ski wax http://www.tokowax.com
This site is jam packed full of great skiing information http://www.skinet.com
Informational site on ski techniques and resorts http://www.breakthroughonskis.com/
Cool site about snow and ice behavior and research http://nsidc.org/index.html
The Ultimate ski links page http://www.geocities.com/Yosemite/9818/