Background Information

The Glugging Theory
Written by Ashley Bonica and Matthew Onhieber
1999-2000

TABLE OF CONTENTS
Background Information
Statement of Problem
Review of Related Literature
Statement of the Hypothesis
Method
Results
Discussion
Graphs
Bibliography
Links
Return to Research

Background Information | Table of Contents

For many years there has been an enigmatic phenomenon that we would like to explain. Why is it that water makes a glugging sound when it is poured from a bottle? This question we will name "the glugging theory", has puzzled physicists for many years. "The glugging theory" has a profound but commonly unnoticed impact on our everyday life. Around the globe, children and adults alike are afflicted with the burdening outcome of the theory. Due to their ignorance of "the glugging theory" they fail to realize the chaotic motion in which liquids are poured. This results in many glasses to be overflowed. Another factor is the high liquid velocity that follows each glug. This factor causes numerous cases of splashing the liquid over the rim of the glass.

P.S. de Laplace made the statement, "regard the present state of the universe as the effect of its preceding state and as the cause of its succeeding state" (McGraw hill). In narrowing this broad statement we realize there must be specific scientific laws causing the previously mentioned phenomenon. The causes we have found are the chaos theory, pressure, and fluid dynamics.

Statement of the Problem | Table of Contents

Our purpose in researching "the glugging theory" is to better understand the phenomenon. We will strive to comprehend all the causes and effects of the theory. In turn, we will be able to warn and educate society in the hope of decreasing the amount of spilt milk and other liquids.

Review of Related Literature | Table of Contents

The results of "the glugging theory" are affected by many variables. The variables that we will experiment on are the affects of the volume of the liquid and the amount of space in which the liquid is allowed to flow out. In order to fully understand the experiments that we are undertaking we must research related topics including fluid dynamics, pressure, and the chaos theory. The chaos theory is related to our own "glugging theory". Vaguely, the chaos theory states that a system’s behavior is random. "The initial conditions of a system are unpredictable and cannot be distinguished from a random process" (McGraw and Hill). This leads us to believe that the glugs of water will not occur in intervals but at random. However, "The glugging theory" will have levels of chaos. According to Kawakami and Funakoshi’s work on the topics of fluid dynamics, fluid particles will move chaotically when the strain rate of the external flow is small. Therefore, the smaller the opening, the more chaotic motion will occur amongst the water particles. Pressure and volume also effect "The glugging theory". Pressure is defined as "force per unit area" (Webster, 905). Pressure in liquid increases with volume. According to this information, we assume when volume increases, the rate of glugging will be faster due to the increase of pressure. On the other hand, when volume decreases the rate of glugging will be slower. In some ways this opposes how the chaos theory relates to our "glugging theory" because it contains order. This is one discrepancy that we will hope to clear by performing our experiments.

Statement of the Hypothesis | Table of Contents

Finally, after researching this intriguing information, we are propelled to further investigate "the glugging theory". "The glugging theory" not only pertains to everyday life; it also has discrepancies that must be cleared up. As researchers, we hypothesize that the more volume of liquid and the smaller the exit, slower intervals of glugging will occur. Similarly, the less volume of liquid and the bigger the exit, the faster the intervals of glugging will occur. We also hypothesize that the only chaos will be the chaotic motion of the water particles exiting the container. The chaos theory will prove false in that there will be a constant rate of glugs, and in this aspect, order will prevail.

Method | Table of Contents

Our experiment was simple to setup. We used a clear PVC tube that had one end closed off completely by duct tape. The other end was covered by one of two caps, one with an opening of area 3.49 cm² and the other with an area of 1.21 cm². We then set up four unique tests: small opening with large volume of water (1500 ml), small opening with small volume of water (750ml) and then big opening with large volume of water, and big opening with small volume of water. We tested our four variables outside. We filled the PVC tube to the right amount of water (either 750 ml or 1500 ml) and then put on one of the two caps on the open end. Then Matt turned the tube upside down and counted the total amount of glugs while Ashley timed. We timed how long it took for the entire tube to drain as well as at what time the first eight glugs occurred. The reason we chose this setup and design is because it was simple and gathered the information we needed. Our first plan was to use various bottles with different size openings and volumes. We realized that this plan would have required many more calculations and there would be too many variables to take into account. We also realized that using one container would be more precise. There is a diagram of this setup and procedure on the following page. We tested each of the four variables three times in order to obtain more accurate results. We recorded all of our data in graph form.

Results | Table of Contents

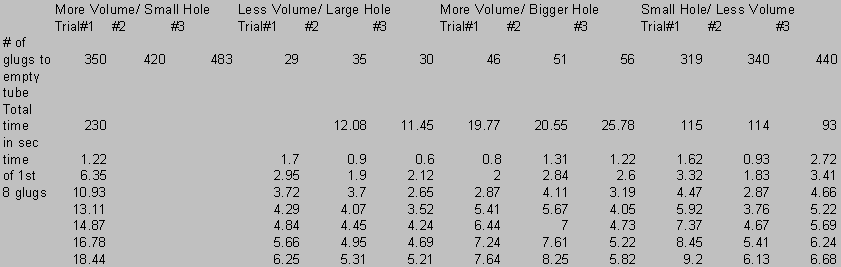
text file - this data in spreadsheet form

The chart above includes all of our data. It is evident that much of the data was not recorded (refer to blank cells). In order to acknowledge these blank spots we averaged the existing data before graphing. Averaging the data from the three trials also made our results more accurate.

Human error proved to be a huge factor in our experiment. It was humanly impossible to count every single glug without missing one. It was literally impossible to see the glugs as they increased to their maximum rate of glugging. Furthermore, the timing could not have been precise due to human reaction time and the inability to visualize each of the first eight glugs. Despite these errors, our graphs were able to show logical results.

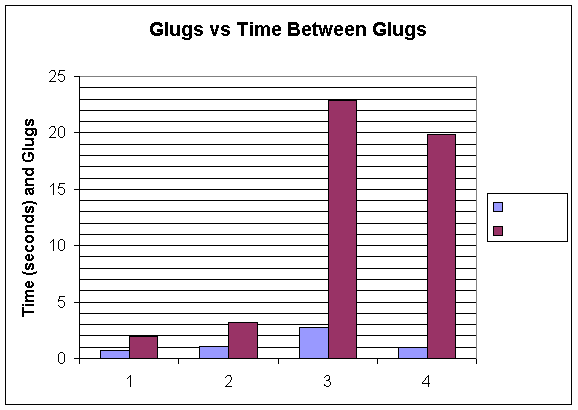
We made six graphs that are on the following pages. The first four graphs (one for each variable) are comparing the time it took the first eight glugs to occur with the difference between these times. We found these differences by subtracting the times of the eight glugs, which we recorded in our chart. The blue line shows that there is nearly a constant rate of glugging, and the pink line shows that the time between glugs is nearly constant, but gets slightly faster as water continues to exit the PVC tube. This is shown by the slight downward slope of the pink line. The pink line is also the derivative of the blue line. After analyzing each of these four graphs it is noticed that in all four the glugs speed up as the water is drained. This is shown because the pink lines generally slope downward. It is also evident that when there is more time involved the glugs start out slower. This is most evident in the graph of more volume/small opening in which the most time was involved (blue line ends around 20 seconds) and the slowest glugs occurred (the pink line starts at 5 seconds). This happens in all graphs although it is less evident. The next graph shows the total number of glugs compared to the total time. In order to graph this we had to find the average amounts of glugs and seconds for each of the four tests. This graph shows the variable that affected our experiment the most to be the size of the hole. In this graph the variables with large holes, regardless of the amount of water involved, had much lower times and glugs involved. The last graph compares the time between glugs to the total amount of glugs. For this graph we had to divide the total amount of glugs by 16 in order for the time difference to show on the page. This division does not effect the validity of the graph because it still shows the differences between glugs correctly. This graph demonstrates the main principal that the more volume, the slower the glugs start out no matter what size the hole is.

Discussion | Table of Contents
Our hypothesis did not hold up to be entirely true. We originally thought that there would be no chaos involved in glugging. In fact there was chaos at the end of each test, especially in those with large openings. Chaotic motion occurred primarily when a larger opening was used and there was a dismal amount of order even when we used the small opening as the water level got low. It was obvious to observe that as the water decreased the glugging rate increased with both the large and small openings, which was not originally hypothesized. This can also be seen in our results shown on the line graphs. Despite the amount of water, the first eight glugs were always the slowest, and even within those eight the glugs began to speed up. This aspect of our experiment proved there was some order. All other parts of our hypothesis turned out to be true.

At the end of our experiment we were not able to determine the causes of glugging. We were, however, able to find what variables create an ideal glugless container. This container would have a low volume and a small opening. It would create extremely fast, small glugs which make a steady stream of liquid. A steady stream is much easier to aim into a glass than the large, slow, chaotic glugs a container with high volume and a large opening would create. As for the effects of glugging we were able to determine that glugs create a stream of liquid that is more likely to spill. The way to avoid a spill is to use the previously mentioned ideal container. Spread the word to be cautious of containers that glug, and think twice when pouring a glass of milk!

For further research, it would be interesting to test various shapes and sizes of containers with different sizes of openings, as we had first planned to do. It would also be interesting to test liquids of higher density than water to see how that would effect the rate of glugging.

Bibliography | Table of Contents

Kuhn, Karl F. Basic Physics: a self teaching guide.

New York, John Wiley and Sons Inc., 1979.

de Laplace, P.S. in McGraw and Hill. McGraw and Hill Encyclopedia of Science: 8^th ed.

New York, McGraw and Hill Inc., 1997.

Funakoshi, Mitsuaki and Kawakami, Akihiko. Fluid Dynamics Research Vol. 25. Pages 167-193. New York, Elsiever Science B.D., 1999.

Weaver, Jefferson Hane. The World of Physics.

New York, Simon and Schuster Inc., 1987.

Webster’s New World Encyclopedia. Elliot, Steven P. ed. "Pressure" p. 905.

New York, Simon and Schuster Inc., 1992.

Links | Table of Contents

http://www.princeton.edu/~gasdyn/fluids.html
This site lists current links to other sites of interests to students and researchers in fluid dynamics. It contains journals and magazines written on fluid dynamics, teachings and lectures on fluid dynamics, projects and experiments in correlation with fluid dynamics, a listing of professional scientific organizations and federal research funding agencies, a listing of key government sites, and individual web page sites on fluid dynamics.

http://www.imho.com/grae/chaos/chaos.html
This site gives a brief introduction to the chaos theory. It also shows graphs of Lorenz’s experiment, the Lorenz attractor the Koch curve, the bifurcation diagram for the population equation, and excerpts from “Does God Play Dice?” by Ian Stewart, and “Chaos – Making a New Science” by James Gleick.

http://physlink.com/ae141.cfm
This is an interactive site that we were able to ask a question which was related to our topic and have it answered by a scientist. Our question was why do you hear a glugging sound when you pour a liquid out of a bottle by holding it upside down? To find the answer just go to this site!

http://www.newswire.ca/releases/June1998/08/c1704.html
This site gives a brief introduction to the chaos theory. It also shows graphs of Lorenz’s experiment, the Lorenz attractor the Koch curve, the bifurcation diagram for the population equation, and excerpts from “Does God Play Dice?” by Ian Stewart, and “Chaos – Making a New Science” by James Gleick.

http://wine1.sb.fsu.edu/chm1045/notes/Forces/Liquids/Forces03.htm
This site contains information on the properties of water as a liquid. Is is on the topic of viscostiy and water tension. These topics apply to our project in that they help explain the motion of the water as it is poured.