James Gardner
How Does the Air Temperature Impact the Frequency of a Guitar String?
Table of Contents .:. Return to Research
Background
The modern standard tuning, or as some may refer to it, ‘concert tuning’, was left untouched for the greater portion of recorded phonic history. Pitch pipes of the Renaissance era all varied slightly in their ideas of proper tuning, and instrumentalist groups found it difficult to correlate their frequencies in any precise manner. The very first tuning fork, invented in 1711 by John Shore, had an entirely arbitrary pitch of A423.5Hz. However, such tuning failed to become the practice standard, and it was not until 1938 that a universal frequency was once again brought up at an international conference. This conference led to the ultimate decision that middle A was to become 440Hz, as advocated by mathematician Sir James Swinburne, and is the expectation today still.
In physics, frequency is the property of a sound wave which determines its pitch. Namely, it is defined as the number of wavelengths, or vibrational cycles, which a sound wave passes through in one second. For example, a sound wave which passes through 200 wavelengths every second has a frequency of 200Hz, and is somewhere between the notes G and G#. Tutorvista’s “Wave Frequency” describes this relationship in the form of the equation f = 1/T, where f is the frequency, 1 is the number of wavelengths, and T is the period of time passed. A smaller period correlates to more wavelengths per second, thus increasing the frequency and pitch. Conversely, a larger period will ultimately decrease the frequency and pitch.
The frequency of a sound wave is additionally dependent on the surrounding air temperature. The NDT Resource Center states that an equation for the speed of sound is given by V = 331m/s + .6T, where V is the speed of sound, and T is the air temperature in Celsius. Furthermore, sengpielaudio’s given correlation that frequency multiplied by wavelength is also equal to the speed of sound shows that a change in air temperature will either affect the wavelength or frequency of the sound. As sengpielaudio’s article describes it, “Since the length of the [instrument] and with it the wavelength λ remain constant, only the frequency f (pitch) will change”(Sengpiel).
Statement
The purpose of this research is to determine the effect that surrounding air temperature has on the frequency of a guitar string.
Hypothesis
My expectation is that an increase in atmospheric temperature will subsequently cause an increase in note frequency, and vice versa.
Variables
The independent variable of this experiment is the air temperature surrounding the guitar string, and the dependent variable is the resulting frequency of the A string on a guitar. Some controlled variables in consideration include the initial frequency of the string, the intensity with which the string is played, and the model of guitar which is utilized.
Relevant Formulas
F=1/T
V=331+.6T
c=fλ
Setup and Procedure
Among the materials that this research will be utilizing are a chromatic tuner, a Carvin acoustic guitar, Keuwlsoft’s “Frequency Counter” phone application, a classic celsius mercury thermometer, and an air conditioning system. The experiment will be conducted within a room of controlled temperature.
To
commence the experiment, the air conditioning system will be set to a
temperature of 50 degrees fahrenheit (10 degrees celsius), and the thermometer will be utilized to more
precisely ensure that 50 degrees Fahrenheit is, in fact, the surrounding air
temperature. WeatherWorks’ article “How to Measure
Temperature Correctly” states that a thermometer will relay the most reliable
temperature information if it is held approximately 5 feet above the ground,
and in a location with good air flow. As such, this will be the subsequent
protocol for handling the thermometer. Once the temperature is established, the
A string of the guitar will be played and held for 3 seconds. This will be
repeated 5 times, and the average frequency of the 5 notes will be recorded
using the frequency counter application. After this has been completed, the air
conditioner will be made to set the room to 68 degrees fahrenheit
(20 degrees celsius) and then 86 degrees fahrenheit (30 degrees celsius),
where the same steps will be completed for each respective temperature.
Figure 1a-
Guitar with Thermometer
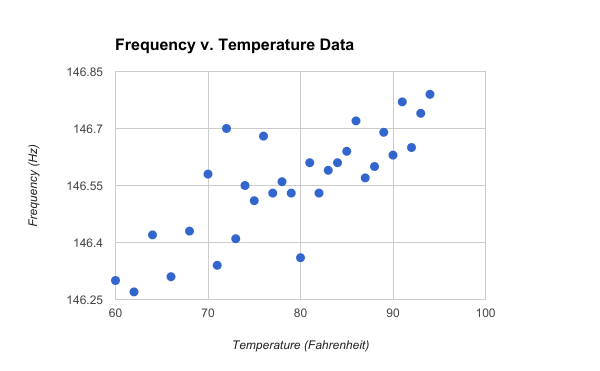
Data and Findings
Within
the investigation, the temperature tested ranged from 60 to 94 degrees
Fahrenheit. The frequency, as such, ranged from 146.27Hz to 146.81Hz.
Data file: text
Conclusion
Due to the data presented, the investigation must ultimately come to the conclusion that air temperature does have an effect on the frequency of a guitar string. Although only very slight, the data followed a loose correlation, indicating that as temperature increases, the frequency increased as well, thus supporting the hypothesis of the investigation. However, my expectation was to see a much larger and more dramatic correlation between the variables. This may be due to the temperature of the string itself, which was not tested. As air does not flow through the instrument itself, as is the case with most woodwinds, the temperature may not have been drastically changed. Another limitation of the inquiry may include the slow and gradual change in tuning which every guitar experiences not as a result of temperature, but of its pitch relative to the other strings. Thirdly, the head of the guitar, which is fashioned with knobs for tuning, may have been unintentionally jostled, affecting the string’s tuning. Finally, the technology which was utilized may have been unreliable, as the frequency counter phone application seemed to have a relatively large range of uncertainty. In a repeated experiment, I would spend more time researching the ideal technologies for this experiment, and perhaps utilize an instrument more conducive to the experiment.
Links
https://www.nde-ed.org/EducationResources/HighSchool/Sound/tempandspeed.htm Explains the relationship between air temperature and the speed at which sound will travel
http://www.sengpielaudio.com/calculator-pitchchange.htm Explains why woodwind instruments will change pitch with air temp changes
https://www.nolapiano.com/blog/2016/7/29/how-do-changes-in-temperature-affect-a-tuning
Very interesting experiment with the piano, a stringed instrument, and how an increase in kinetic energy will massively affect the instrument’s tuning
https://www.youtube.com/watch?v=baenrE6yVlY Comedic video about the history of pitch and what is acceptable tuning
http://www.theconcertband.com/index.php/resources/music-physics/pitch-and-temperature Information about how temperature can have a real life impact on musicians
Bibliography
"Temperature and the Speed of Sound." NDT Resource Center. N.p., n.d. Web.
Sengpiel, Eberhard. "Change of Pitch With Change of Temperature." Sengpielaudio. N.p., n.d. Web.
"Wave Frequency." TutorVista. N.p., n.d. Web.
"Standard Pitch or Concert Pitch for Pianos." History of Pitch - Tuning Forks A440 C523.3. N.p., n.d. Web.
"Speed of Sound in Air." Hyper Physics. N.p., n.d. Web.
"How to Measure Temperature Correctly." WeatherWorks. N.p., n.d. Web. 23 Nov. 2016.