Air Resistance: The Effect on a Falling Ping Pong Ball’s Velocity and Energy
Researched by Teagan Goforth
Physics Higher Level
March 2016
It is common knowledge that a parachute is needed in order to reach the earth safely if one is falling through the air, like in skydiving, but what is not common knowledge is the why. Why would a skydiver hit the earth at a very high speed if he did not have a parachute? The reason is air resistance. If a skydiver did not have a parachute, there would be a very small force of air resistance and a very large force of gravity pulling him to the earth (“Air Resistance Force”). Air resistance, or air friction, is a force that acts on objects falling through the air. It is a type of frictional force, which is a force that is opposes the object’s direction of movement. Air resistance is due to collisions among molecules and the object. It is dependent on two variables: the surface area and the speed of the falling object (“Free Fall and Air Resistance”). The larger the surface area of the object and the faster the object is falling, the the larger its air resistance will be. As the object falls for a longer period of time, its air resistance will increase due to its increasing velocity. Because of this, it is possible for an object to eventually reach its terminal velocity, meaning it cannot accelerate any faster than this particular velocity. The terminal velocity of an object is reached when its air resistance force equals the force of gravity, resulting in a net force of zero (“Free Fall and Air Resistance”).
Although air resistance is negligible in many circumstances, it is still important to take into account. Galileo made a hypothesis that anywhere on earth, two objects would fall with the same constant acceleration in the absence of air resistance, emphasizing that air resistance does impact two object’s ability to fall at the same acceleration. For example, a feather and a rock are put in a chamber with air and a chamber in which the air has been vacuumed out. In the chamber with the air, the feather will take longer to fall to the ground than the rock, but in the vacuum chamber, the two objects will fall at the same acceleration (Giancoli). The feather falls slower than the rock because the feather reaches its terminal velocity, where the force of gravity and air resistance are equal, faster than the rock does due to the difference in mass (“Elephant and Feather - Air Resistance”). However, calculating air resistance analytically can be a very complicated process (“Air Friction”). Air resistance’s impact on energy has to do with the potential and kinetic energy of the falling object. More air resistance will result in less kinetic energy. I am very interested to find out just how much air resistance has an impact on calculations because we usually ignore it while doing labs or theoretical calculations.
The purpose of this investigation is to determine how the height of a falling object has an effect on the object’s energy that is lost to air resistance. The independent variable is the height from which the ping pong ball is dropped, and the dependent variable is the amount of energy lost.
I believe that the higher the object is falling from, the more air resistance it will accumulate and thus, the more energy it will lose. This will happen because the more time an object has to fall to the ground, the more its velocity will increase, and air resistance is dependent on velocity. Therefore it causes the air resistance and energy lost to also increase.
To
test this idea of air resistance and energy lost, I dropped a ping pong ball
from varying heights and measured its velocity and energy. The mass of the ping
pong ball is 2.7 grams. The heights I dropped the ping pong ball from were 0.5
feet (0.1524 m), 1 foot (0.3048 m), 1.5 feet (0.4572 m), 2 feet (0.6096 m), 2.5
feet (0.7620 m), 3 feet (0.9144 m), 3.5 feet (1.0668 m), and 4 feet (1.2192 m).
Once I had the velocity, I was able to input the values into the equation ![]() to find the actual energy of
the ping pong ball and compared it to its theoretical energy, which I
calculated using
to find the actual energy of
the ping pong ball and compared it to its theoretical energy, which I
calculated using ![]() .
.
Diagram of set up:

First, to find the velocity, I used the program LoggerPro and attached two photogates to it. The photogates were assembled right above the ground in order to find the velocity of the ping pong ball as it was about to hit the ground. Being set up one on top of the other, the photogates were able to calculate the velocity through the distance they were set apart, 5 centimeters, and the amount of time it took to pass through one and then the other. The equation that LoggerPro used to calculate its velocity was 0.05/BlockedToBlocked("Time", "GateState 1", "GateState 2"). I dropped the ping pong ball from each height 20 times. Also, there was a small pillow placed underneath the photogates in order to ensure that the ping pong ball would not bounce back up through the photogates.
Then,
using the values given to me from LoggerPro, I was
able to calculate what the actual energy of the ping pong ball was versus what
the expected energy of the ping pong ball was. I then used the equation ![]() to find the total amount of
energy lost due to air resistance.
to find the total amount of
energy lost due to air resistance.
The data collected shows that the both the velocity and energy lost increase linearly based on the height it was dropped from. The higher the ball was dropped from, the faster its velocity, so the greater air resistance it accumulated. The average velocity of each height increased at a rate of 3.008 m/s/m. The expected energy increased at a rate of 0.026 J/m, while the calculated energy only increased at a rate of 0.022 J/m. Although the rate of change of kinetic and potential energy are almost equal, kinetic energy was always less than the potential energy calculated, and this is because of the air resistance that the ping pong ball faced while falling. And finally, the energy lost increased at a rate of 0.00424 J/m. All of the data points collected resembled that of a line, and were able to be plotted on a linear trendline with a high correlation value.
The uncertainties were calculated by using the equation: uncertainty = range/2.
Table 1: Average Velocities
|
Height |
Average Velocity +/- 2 m/s |
|
0.5 feet (0.1524 m) |
0.832276809 m/s |
|
1 foot (0.3048 m) |
1.995233167 m/s |
|
1.5 feet (0.4572 m) |
2.482999245 m/s |
|
2 feet (0.6096 m) |
2.972994513 m/s |
|
2.5 feet (0.7620 m) |
3.389841354 m/s |
|
3 feet (0.9144 m) |
3.663471811 m/s |
|
3.5 feet (1.0668 m) |
4.136180300 m/s |
|
4 feet (1.2192 m) |
4.238508298 m/s |
Figure 1: Velocity vs. Height Graph
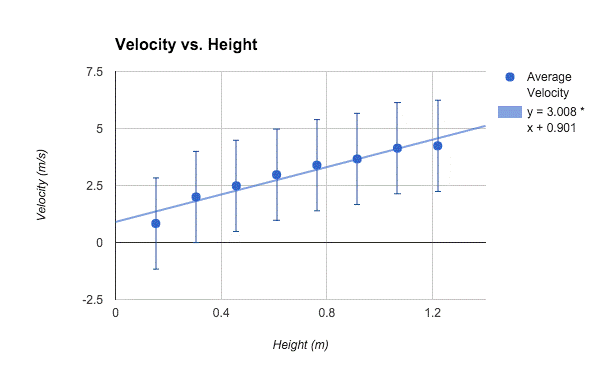
Table 1: Expected energy
|
Height |
Potential
Energy ( |
|
0.5 feet (0.1524 m) |
0.0027 * 9.81 * 0.1524 = 0.00403662 J |
|
1
foot (0.3048 m) |
|
|
1.5 feet (0.4572 m) |
0.0027 * 9.81 * 0.4572 = 0.01210986 J |
|
2
feet (0.6096 m) |
|
|
2.5 feet (0.7620 m) |
0.0027 * 9.81 * 0.7620 = 0.02018309 J |
|
3
feet (0.9144 m) |
|
|
3.5 feet (1.0668 m) |
0.0027 * 9.81 * 1.0668 = 0.02825633 J |
|
4
feet (1.2192 m) |
|
Table 3: Calculated Energy
|
Height |
Kinetic
Energy ( |
|
0.5 feet (0.1524 m) |
0.5 * 0.0027 kg * (0.832276809 m/s)2 = 0.00093512 J |
|
1 foot (0.3048 m) |
0.5 * 0.0027 kg * (1.995233167 m/s)2 = 0.00537429 J |
|
1.5 feet (0.4572 m) |
0.5 * 0.0027 kg * (2.482999245 m/s)2 = 0.00832314 J |
|
2 feet (0.6096 m) |
0.5 * 0.0027 kg * (2.972994513 m/s)2 = 0.01193224 J |
|
2.5 feet (0.7620 m) |
0.5 * 0.0027 kg * (3.389841354 m/s)2 = 0.01551288 J |
|
3 feet (0.9144 m) |
0.5 * 0.0027 kg * (3.663471811 m/s)2 = 0.01811838 J |
|
3.5 feet (1.0668 m) |
0.5 * 0.0027 kg * (4.136180300 m/s)2 = 0.02309578 J |
|
4 feet (1.2192 m) |
0.5 * 0.0027 kg * (4.238508298 m/s)2 = 0.02425268 J |
Figure 2: Potential Energy vs. Kinetic Energy Graph
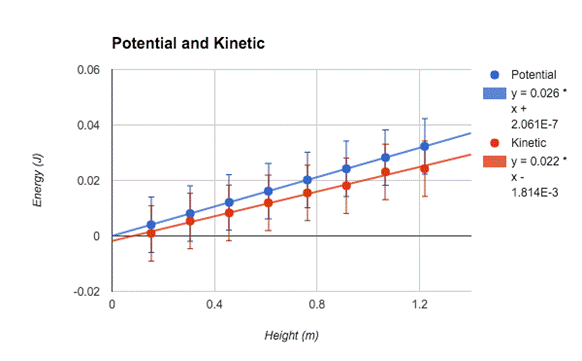
Table 4: Total Energy Lost to Air Resistance
|
Height |
Total
Energy Lost ( |
|
0.5 feet (0.1524 m) |
0.00403662 J - 0.00093512 J = 0.00310150 J |
|
1 foot (0.3048 m) |
0.00807376 J - 0.00537429 J = 0.00269947 J |
|
1.5 feet (0.4572 m) |
0.01210986 J - 0.00832314 J = 0.00378672 J |
|
2 feet (0.6096 m) |
0.01614647 J - 0.01193224 J = 0.00421423 J |
|
2.5 feet (0.7620 m) |
0.02018309 J - 0.01551288 J = 0.00467021 J |
|
3 feet (0.9144 m) |
0.02421971 J - 0.01811838 J = 0.00610133 J |
|
3.5 feet (1.0668 m) |
0.02825633 J - 0.02309578 J = 0.00516055 J |
|
4 feet (1.2192 m) |
0.03229295 J - 0.02425268 J = 0.00804027 J |
Figure 3: Energy vs. Height Graph
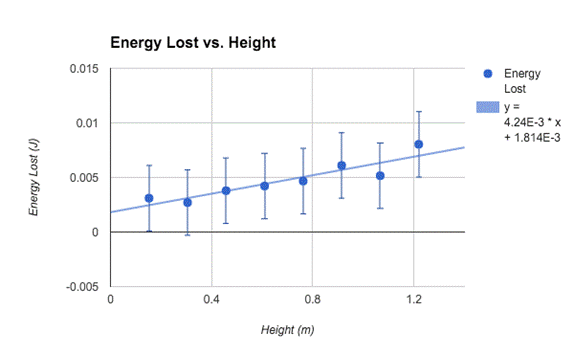
My hypothesis of this experiment was that the higher the ping pong ball fell from, the more energy it would lose to air resistance.The data supports this hypothesis because the higher it was dropped from, the higher the velocity, and thus, the higher the energy lost to air resistance. I think it is very interesting that the energy lost increases linearly based on the height it was dropped from. For further research, it would be interesting to see if the ping pong ball’s linear trend of energy lost continues for higher heights. Also, it would be interesting to test how long it takes an object to reach its terminal velocity, and therefore its highest possible air resistance.
There are multiple sources of error in this experiment. First of all, due to human error, the ping pong ball may not have been dropped precisely at the given heights. Also, the velocity of the ping pong ball may not have been exactly accurate. That is why 20 trials were done for each height, and the average velocity was taken. Changes that could be made to this experiment would be to possibly set up a platform at each height so it is dropped from the exact same place every time. Another source of error is the way the ping pong ball was dropped through the photogates. Sometimes the ping pong ball would bounce off a photogate and still pass through the second one, leading to the calculation of a skewed data point. I did attempt to identify these data points and not use them in my calculations, but it is possible that a few remained.
Although there were some sources of error, the uncertainties were very limited and the data points seem to be rather accurate. Therefore, this experiment supported my hypothesis of increasing air resistance as the height increased as well. Although air resistance is usually negligible while doing calculations, it is important to take into account that it will make an effect on one’s data points while doing an experiment.
http://hyperphysics.phy-astr.gsu.edu/hbase/airfri.html
This website mainly just highlights the equations that are used to estimate air
friction. I found it helpful in describing and determining the variables.
http://www.skwirk.com/p-c_s-11_u-399_t-988_c-3779/air-resistance-force/nsw/science-technology/forces-and-their-effects/types-of-forces
This page is interesting because it explains the different types of forces that
are acting on a falling object, as well as what air resistance is.
http://www.physicsclassroom.com/mmedia/newtlaws/efff.cfm
I like this page because it explains why two objects of different masses will
fall at the same rate.
http://www.physicsclassroom.com/class/newtlaws/Lesson-3/Free-Fall-and-Air-Resistance
This website gives the basics of what free fall is and how air resistance
accumulates. It also offers some riveting free fall calculations to do.
"Air Friction." Hyperphysics. N.p., n.d. Web. 28 Nov. 2015.
"Air Resistance Force." Skwirk Online Education. Red Apple Education Ltd, n.d. Web. 28 Nov. 2015.
"The Elephant and The Feather - with Air Resistance." The Physics Classroom. N.p., n.d. Web. 28 Nov. 2015.
"Free Fall and Air Resistance." The Physics Classroom. N.p., n.d. Web. 28 Nov. 2015.
Giancoli, Douglas C. Physics: Principles with Applications. 6th ed. Upper Saddle River, NJ: Prentice Hall, 2009. Print.