Data
Solving for Ending Velocity, right? (since muzzle velocity is assumed to be
the same every time).
I'll give you five bucks if you can decipher my RAW data:
Horiz. Displace (m) |
Vert. Displace (m) |
Calculated Muzzle Velocity |
Average Velocities |
|
0.1600 |
0.0239 |
186.1634 |
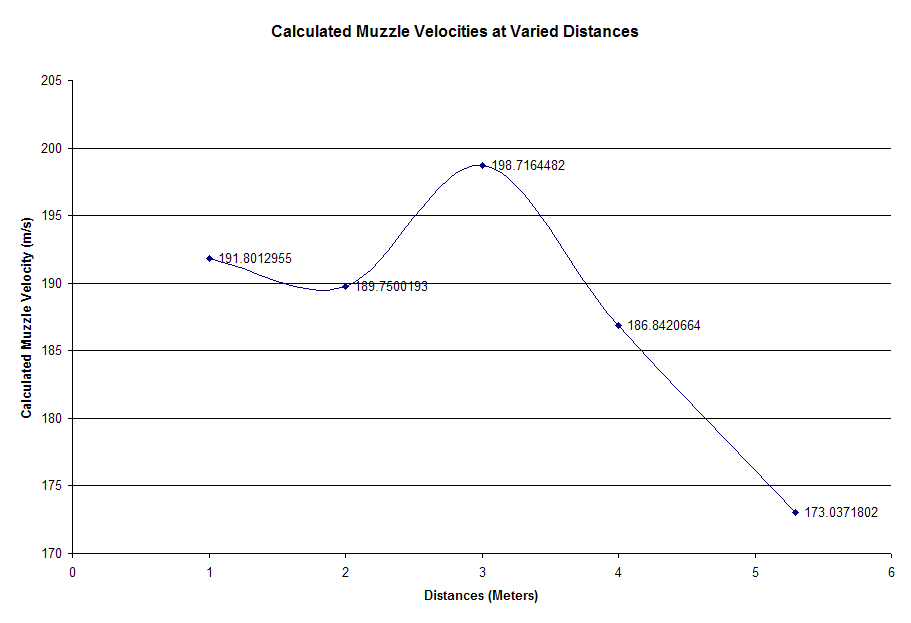
191.8013 |
|
0.1550 |
0.0224 |
180.8820 |
(+ or -) 14.84 |
|
1 Meter |
0.1550 |
0.0224 |
181.5443 |
|
0.1675 |
0.0262 |
197.2545 |
||
0.1800 |
0.0304 |
213.1623 |
||
0.1700 |
0.0270 |
201.7302 |
189.7500 |
|
0.1600 |
0.0239 |
190.2681 |
(+ or -) 14.68 |
|
2 Meters |
0.1400 |
0.0182 |
166.6413 |
|
0.1600 |
0.0239 |
191.6363 |
||
0.1650 |
0.0254 |
198.4742 |
||
0.1650 |
0.0254 |
199.1802 |
198.7164 |
|
0.1675 |
0.0262 |
202.9902 |
(+ or -) 15.38 |
|
3 Meters |
0.1600 |
0.0239 |
194.3727 |
|
0.1650 |
0.0254 |
201.2982 |
||
0.1600 |
0.0239 |
195.7410 |
||
0.1490 |
0.0206 |
182.6457 |
186.8421 |
|
0.1500 |
0.0209 |
184.5362 |
(+ or -) 14.46 |
|
4 Meters |
0.1425 |
0.0189 |
175.7482 |
|
0.1600 |
0.0239 |
198.4774 |
||
0.1550 |
0.0224 |
192.8028 |
||
0.1390 |
0.0179 |
173.1356 |
173.0372 |
|
0.1510 |
0.0212 |
189.0153 |
(+ or -) 13.39 |
|
5.3 Meters |
0.1300 |
0.0156 |
162.8620 |
|
0.1350 |
0.0169 |
169.7988 |
||
0.1350 |
0.0169 |
170.3742 |
Uncertainty = (0.00001/0.00032+0.001/0.548+0.00025/0.08676+0.1/9.8+0.005/0.16)
Mass of target Before (kg) = 0.0858
Mass of target After (kg) = 0.0950
Mass of BB (kg) = 0.00032
Radius (meters) = 0.5480
I first used the Observed Horizontal Displacement to calculate the Vertical Displacement. Formula:
Vertical Displacement = (Radius)-((((Radius)^2)-((Observed Horizontal Displacement)^2))^(1/2))
Then I used that Vertical Displacement to solve for Velocities for each trial:
Velocity = ((Mass of Target Before)+((Mass of BB)*(Number of Times you've shot the target previously))*(2*9.8*(Vertical Change))^(1/2))/(Mass of BB)
Tricky.
Download the Data here (right click, save as... or just click it to open in a new window):
Graph