Justin Crandall and Todd Glazier
Introduction Method Launch Pad Rocket Design
Results Graph Discussion Disclaimer Sources
By simply looking at water rockets, the elements involved appear to be quite elementary, in fact, water rockets was an experiment we did in 5th grade. You put water in the rocket, apply pressure to the inside of the bottle, release it and off it goes. Unfortunately for us, we have a high enough understanding that there is a lot more to water rockets than this. Such elements include angle, effective construction of bottle to achieve maximum height, amount of water, and of course the amount of pressure given to the bottle. Given this we set out to determine at what volume of water is the highest velocity achieved with a constant pressure applied.
The basic components of a bottle rocket for the most part are very simple. As I said above, angle, construction, water and pressure are the key elements for the launching of a water rocket. Compressing the air inside the bottle with any given amount of water exerts a force on the bottle that creates a scenario in which the bottle, when released, will release the water which flows out of the rocket pushing the rocket into the air. Now if we look to Newton’s third law, “Whenever one object exerts a force on a second object, the second object exerts an equal and opposite force on the first object” (Giancoli 83). This means that the water thrust given by the rocket inversely corresponds to the rocket elevating upward. We already know from Newton’s second law of motion that F=ma and the two forces (the thrust and that of the water rocket) should be equal (81). Newton’s laws are essential in understating the physics applicable to the water rockets we are studying, the more force on an object the more it accelerates, but the more massive it is the more it resists acceleration (http://woodmansee.com/science/rocket/r-other/rb-equations.html). Usually the mass is constant when using F=ma, but with rockets the mass changes because of the expulsion of the water shortly after takeoff. This meant we could not use this formula in exactly the same way as it is stated. We learned that the exhaust velocity is constant, so the mass flow rate time the exhaust velocity equals the force. Pressure is also related to force because the pressure times the area it’s applied against gives the extra force (http://woodmansee.com/science/rocket/r-other/rb-equations.html). This was just the preliminary problems, but we will further pursue these equations later when we discuss our results.
There were other sites that became informative and helpful in our quest to reach the highest velocity of a water rocket. We found that the best way to find information pertaining to our study was on the internet considering not many books are published on the subject of bottle rockets. The fist site we found in our search for information was http://ourworld.compuserve.com/homepages/pagrosse/h2oRocketIndex.htm which was helpful in the construction phase of our project but did not lend itself to much else. The second website, http://hometown.aol.com/hayhurst1/h2orocket.htm, helped us when looking for possible questions to answer. Lastly, before we acquired our own launcher, http://home.nc.rr.com/enloephysics/enloephysics/Rocket/Page_1x.html gave us some basic ideas for how to propel our bottle rocket skyward. We also found some simulations that we couldn’t find useful but, nevertheless we derived hours of enjoyment from them (http://water-rockets.com/javasim/index.html).
Now that we have constructed a foundation upon which an understanding of water rocketry, we must form a research question in which our project will be focused around. At what volume can a maximum velocity be reached, in a 2 liter bottle, by utilizing water bottles and applying a constant pressure of 70 psi to the water rocket? We hypothesize that applying approximately 70 psi to the water rocket filled with 1 liter of water will ultimately achieve the greatest velocity. Our reason for coming to this theory is because filling the bottle with 1 liter will create a .5-.5 ratio of water to air thus allowing a great amount of pressure to be placed in the rocket while not over weighing it permitting a high velocity. Top of Page
Method: Firstly we needed a launch pad, our options were buy one or make one, either way would require effort, time, and brains (none of which we have), so as we discussed said topic with our high and mighty physics teacher, he let us in on a little known secret, “I have a launch pad you could use”. It appears a few years back an elder of the physics clan had acquired a launch pad for a similar project involving water rocketry. They bought this launch pad from Versey Enterprises and when done, donated it to the Physics class for the future of science progression. This is a diagram of the launch pad.
The above launch pad was a very simple design. There is a main PVC pipe that is directly connected to a bike pump hose converter. Along the way there is a handy pressure gauge that goes up to 120 psi. All of this is assembled in a plastic stand that is secured to a heavy wooden base. The wood base serves mainly as a support to prevent any uncertainty in the launch from wobbling. The piping then continues along a curved joint that the goes directly up onto the main launch site. Here there is an O-ring that is the right size so that the nozzle can connect directly onto it, and is then secured by a metal prong. The O-ring is constructed of a rubber ring as to create an air tight seal with the bottle and the stand. This allows the pressure we bring the bottle up to, to remain constant giving us more exact results leaving little uncertainty. Then, the metal prong goes through both sides of the O-ring and just above the ridge of the nozzle thus securing it into place so it does not happen to go off mid-pump. Attached to the end of the metal prong is a piece of rope that is about 10 feet long. This is one of our many safety precautions, allowing us plenty of room to back away eliminating chances of injury. To catapult the rocket all one has to do is give the rope a slight tug which releases the metal prong fastener and allows for the pressure to propel the rocket away from the launch pad.
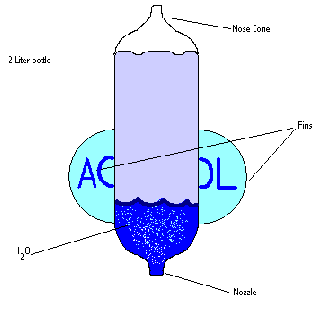
As far as rocket construction is goes, our rocket consisted of a 2 liter bottle with a rounded funnel duct taped to the bottom of the bottle. This served as a nose cone that mainly added to the aerodynamics of the rocket allowing greater velocities and heights. Unfortunately during a few of our experiments, many funnels were lost in the line of duty and so we quickly learned how to construct more efficient rockets that broke less and flew better. For fins we duct taped on halves of AOL CDs (their light, cheap yet solid frame created optimal steadiness during flight) and experimented with both 4 and 3 fins and eventually decided on 3 fins, mainly because it eliminated from the overall weight of the bottle, yet still contributed to the steadying of the rocket.
The next process in the experiment was to find an open field we could use for our rocket launching free from any life which could be threatened due to our negligence. We found this in a field behind our church where there are few things for us to harm. We filled a cooler with all the water we thought we would need for the day considering the amount of trials we intended. We used a video camera to tape our shenanigans for posterity and also for the purpose of the physics symposium to indoctrinate our peers and parents. This being said we got all bundled up trying to compensate for the cool December morning/afternoon for which we scheduled our experimenting. We did repeated trials and copied the results down in a log book.
We picked fourteen amounts of water with which to test with our constant pressure of 70 psi. In our preliminary data we experimented with multiple pressures, but at the advisement of our omniscient instructor Mr. Murray we chose a constant pressure. The selection of 70 psi as the pressure was a lot less scientific than perhaps it should have been, but 60 psi was too small because it did give as much air time and 80 psi was too high because it was hard to obtain with the smaller amounts of water. The amounts of water we selected are as follows: .1L, .2L, .3L, .4L, .5L, .55L, .6L, .65L, .7L, .75L, .9L, 1L, 1.5L, and 2L. To measure the time we used a hand-held stopwatch and made the person who pulled the rope start the clock simultaneously with the launch of the rocket and follow its path to the ground, hopefully ensuring us more accurate results.
Results: Here is a table of the data points we measured.
|
Liters (L) |
Pressure (psi) |
Trial 1 (s) |
Trial 2 (s) |
Trial 3 (s) |
Trial avg. (s) |
|
|
0.1 |
70 |
4.13 |
4.02 |
4.21 |
4.12 |
|
|
0.2 |
70 |
4.43 |
4.38 |
4.33 |
4.38 |
|
|
0.3 |
70 |
4.57 |
4.42 |
4.46 |
4.48 |
|
|
0.4 |
70 |
4.46 |
4.5 |
4.75 |
4.57 |
|
|
0.5 |
70 |
4.83 |
4.86 |
4.77 |
4.82 |
|
|
0.55 |
70 |
4.97 |
5.01 |
5.12 |
5.03 |
|
|
0.6 |
70 |
5.24 |
5.16 |
5.3 |
5.23 |
|
|
0.65 |
70 |
5.09 |
4.95 |
4.87 |
4.97 |
|
|
0.7 |
70 |
5.02 |
5 |
4.83 |
4.95 |
|
|
0.75 |
70 |
4.68 |
4.71 |
4.62 |
4.67 |
|
|
0.9 |
70 |
4.58 |
4.49 |
4.4 |
4.49 |
|
|
1 |
70 |
4.54 |
4.37 |
4.33 |
4.41 |
|
|
1.5 |
70 |
3.71 |
3.97 |
3.88 |
3.85 |
|
|
2 |
70 |
2.29 |
2.12 |
2.22 |
2.21 |
|
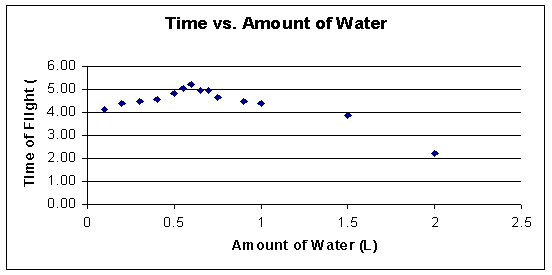
The first column is equal to the amount of water we put in the 2 liter bottle prior to liftoff. The second is the constant pressure we acquired by pumping and reading the pressure gauge contained on the launch pad. The following three columns are for each of the separate trials we performed concluding with the average. This is a graph of the average time of flight and the amount of water.
After doing these easier parts we had to reach deep into our closets and get our scientific hats on, which proved to be a little small. We originally set out to find the initial velocity and reached numerous roadblocks, but nevertheless we trudged onward and tried to explain the mysteries of sciences (or at least mysteries to us). By looking back at Newton’s first law which essentially states that an object at rest tends to stay at rest and there is a necessary force to change that velocity (Giancoli 78). When using the equation F=ma, the mass is constant, but with bottle rockets the mass changes after liftoff when then water mass is forced from the bottom. With this changing mass it would be inaccurate to calculate using this exact equation. However, there is an equation that pertains to the exhaust products of rockets. By understanding this concept we see that the mass isn’t constant, but changes in relation to the exit velocity of the fuel (water). This number is a constant and we are able to substitute this with the mass flow rate to find the force. The mass flow rate is the mass of the fuel divided the time it took to expend it all. This gives us the new formula F= (mass of fuel/time to expend) x exhaust velocity (Ve). This gives us the thrust of the rocket at a given time; however, it ignores any external elements that might affect it. One particular aspect of the rocket that we are neglecting is the matter of how pressure applies force. A formula we found, F=(mass flow rate)( Ve) + Ae (Pe-Pa), was provided by http://woodmansee.com/science/rocket/r-other/rb-equations.html, a water rocket based website. Where Ae=area of the exit plane (nozzle), Pe=rocket exhaust pressure at the nozzle, Pa=outside atmosphere pressure. Woodmansee put it like this, “When the nozzle pressure is higher than the outside atmospheric pressure, the exhaust area is too small, and not all the energy has been converted to exhaust velocity so the thrust isn’t optimized. Alternatively, when the nozzle pressure is lower than the outside atmospheric pressure then the second term becomes negative and actually causes drag instead of a thrusting force.” By finding the thrust we hoped to be able to use that in calculating the exhaust flow rate which would be beneficial later as we will explain. Now looking at the above equations, we have known values for many parts of the equations. Applying these parts of the equations, mass of fuel, time to expend, area of nozzle, outside pressure, and exhaust pressure at nozzle, we can hypothetically find results for our data. The following are our known data values.
Total mass of the rocket = mass of the water + .125 kg
Mass of water = amount of water converted kg, (1 L = 1 kg)
Time to expend fuel = approx.75 seconds
Area of nozzle = π 2.54cm2
Outside pressure = 1 atm = 14.7 psi
Exhaust pressure at nozzle = 70 psi
Now you’re probably asking yourself, what does all this accomplish? We’ll tell you. With the flow rate and the exit velocity being constants, it is safe to say that the thrust is constant. Now going back to our original equation, F=mass flow rate (m*) Ve and m (a) we can substitute one for F and reach m (a) = (m*) Ve solving for a gives us a= m* Ve / m. When using all this we set out to find the change in velocity (dV) which is basically equal to the initial velocity, the change in velocity being from zero until the velocity at which it took flight. We then derived the equation F= d/dt(mV) and would like to thank Woodmansee again for his calculus powers beyond our comprehension to integrate the formula dV= Ve ln(mo/mf). Where delta V is equal to the exhaust velocity times the natural log of the initial mass of the rocket divided by the final mass.
This all sounded great until we realized that we had too many unknowns to solve the equations i.e., dV and Ve. If we had been able to find either the force, the change in velocity, or the exhaust velocity we would have been in 7th heaven, but instead we ended up in the 7th ring of hell. We would have been able to take the necessary steps to solve for the initial velocity. We tried additional research on this topic but only to come up empty handed and without a scientifically confirmed answer to our hypothesis. Top of Page
Discussion: Our original hypothesis that one liter of water would amount to the greatest initial velocity was never fully tested. We feel that with this experiment we did accomplish some good. When we first set out to do our experiment, we felt little hesitation as far as our ability to succeed in finding the desired solution. We were sure that we had the correct formulas, setup and tools necessary to accomplish this goal. Once we recorded our preliminary data, we felt very confident about what we would be able to attain. We have come a long ways since that point. At that time we treated this as a free fall velocity problem disregarding the upward thrust of the water coming out of the rocket, therefore recklessly abandoning the notion that maybe we might need that thrust. Fortunately after presenting our working set-up, our instructor kindly informed us that we were determining the incorrect initial velocity and that there was another way to do it.
We made the mistake of assuming we would be able to figure it out on our own with our inadequate knowledge of the subject. Resulting from this were long, agonizing hours of many attempts to create or manipulate formulas that might result in our success. The closest we got to attaining an answer to our research question was through experimental means rather formulaic which, unfortunately, cannot be backed up by laws, only by theories. We feel that based upon these assumptions the greatest initial velocity was ultimately reached by .6 L and 70 psi. We believe this because upon observations, this launch remained in the air the longest amount of time for all three trials.
We also acknowledge that this disproves our hypothesis that 1 L of water is the optimal amount of water required to reach the greatest initial velocity. We would have liked to prove or disprove our hypothesis using formulas, but sadly enough, we had to result to observational methods to arrive at our conclusion. Now that we recognize the fact that our initial hypothesis was incorrect, we create a new hypothesis. We now hypothesize that this point of optimal initial velocity is at .6 L and we are now only waiting to prove/disprove that using formulas.
Now looking at the graph we provided above, it is obvious that there is a correlation to total weight of the bottle (including water) and the time the bottle spends in air. There is an apparent point at which the time stops increasing and begins to decline again, going to our lowest time of 2.29 seconds with 2 L of water. The least amount of water we used was .1 L and this showed to be just minimally less effective than .6 L compared to 2 L. This shows that because of the immense weight of the bottle when it is fully filled (as well as the lack of air necessary for air pressure), there is a noticeable effect on the rockets ability to maintain a high initial velocity. While at .1 L, there is a .05-.95 ratio of water to air, allowing more room for air pressure to give the rocket propulsion while not weighing it down with excess water. This, however, did not give enough water for the air to exert its force against resulting in less air time. Although when the 2 L bottle was filed with 2L of water there was no room for the air pressure to act as propellant. We suppose that we found the correct balance of water to air ratio at .3 to .7, which amounts to .6 L of water and 1.4 L of air.
One of the inherent aspects of science is uncertainties, which are included even in water rocketry. A few of the key uncertainties involved in our project are precision of timing, measurement of air pressure/water amounts, neglecting of external environmental factors such as wind, and most obviously human error. Firstly, the accuracy of our timer is obvious because there are only a few ways to achieve a truly pinpoint measurement of time; our estimate is +/-.05 s. For the measuring of our water amounts we used a standard 1 L kitchen liquid measuring cup, which sometimes made it hard to narrow down the exact amount of water that we would be filling the rocket with (the surface we set it on may not have always been level either); our estimate +/- .01 L. One minor error we noticed was a small leak coming from the launch pad. When we noticed this we attempted to compensate for it by overshooting the target of 70 psi, and then would let it settle to our desired pressure. But for the most part, the pressure gauge tended to be mostly accurate; our estimate +/- 2 psi. There was no apparent wind at the time of launching the rockets, however there was little we could have done to eliminate this factor, had it existed, as well as possible weather patterns. Overall, we noticed there to be little to none uncertainties in our experiment.
So what kind of other elements of rocketry could we have conducted tests with? What if we had used 1 L bottles instead of 2 L, would we have been able to achieve a greater height or even greater initial velocity? How would varying nose cones have influenced the way in which the rocket flew, including a weighted cone or different materials or shape? How would the viscosity of the liquid have some bearing on the amount of pressure exerted? The final point that may have had an effect on the rockets flight was the size/shape/material of the fins, due to the fact that they impacted the ability of the rocket to remain in a straight flight upward. Top of Page
http://tuhsphysics.ttsd.k12.or.us/Research/ib01/SpinGlaz/index.htm
This website was actually created by physics students from 2 years ago who performed a similar experiment
dealing with water rockets. The website itself gave us the idea, but they purchased a launch pad which we were
able to use in our research project.
http://woodmansee.com/science/rocket/r-other/rb-equations.html
From this site we received crucial formulas pertaining to the science of water rocketry. The main topic was
Conservation of Momentum and one example of a formula is m1+m2+m3...= 0. The only thing that was lacking
that would have aided our research was application of the information in terms of how he used these formulae.
http://water-rockets.com/javasim/index.html
This was a applet that simulated a rocket being launched and gave the results (initial and final velocity, distance)
depending on the specifications on amount of water, weight, and size of bottle. While we actually did follow through
the launching of rockets, we were able to get an approximate comparison to our results after we had completed the
launching process
http://ourworld.compuserve.com/homepages/pagrosse/h2oRocketIndex.htm
The only semi-useful information we gained from this site was the general construction necessities for a the launch
pad. Since we already had a launch pad, it didn't accomplish much, so in the end we were just able to learn why our
pad was built the way it was.
http://hometown.aol.com/hayhurst1/h2orocket.htm
Rocket construction was made available by this site as it gave us ideas for how the fins/nose/bottle could be formed
in order to achieve our desired results. This site was aimed towards more serious water rocket scientists, so some of
the ideas he gave (parachutes/long distance launching pads) were above our heads, so we just stuck with the more
simple designs.
*Disclaimer: While we would like to thank you for
indulging us with your precious time and submitting yourself to this most
incoherent babbling that we consider to resemble a well thought out physics
research paper, we disavow ourselves from any potential harm this may have
caused you