Introduction
Use of combustible material to launch or propel objects has been around for quite some time. Back in the medieval times, people used combustible powders, such as black powder or gunpowder, to launch a cannon ball at a high rate of speed to inflict damage on enemies. Today this has been greatly refined to an art. This practice has evolved into the propulsion of vegetables in what we know as a potato cannon. One main difference between cannons of old and the current potato cannon is the material used to create the force. Potato cannons typically used by high school students involve hair spray or similar substances. Potato cannons aren't used for destruction (mostly); instead they are used for entertainment purposes. But they also have a lot to do with physics. By use of combustion through a focused point, potato cannons propel objects at a high velocity. The question at hand, is how fast exactly can they shoot? This is a question that we are determined to answer. By use of physics principles we plan on determining the initial velocity of potatoes launched, the height reached by the potato when fired straight up, as well as the total energy present in the system. Our hypothesis is that from David Thalers house we will be able to hit the residence of one Tony Ding.

Return to Table of Contents

Method
1. Commandeer a potato cannon.
2. Obtain a bag of potatoes and a bottle of hair spray.
3. Mass potato readied for launch
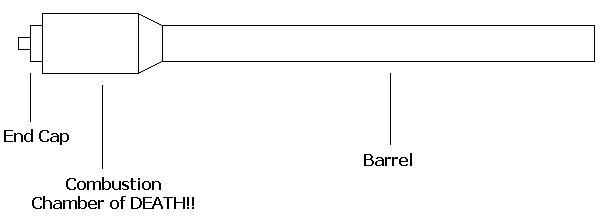
4. Prepare potatoes for launch by stuff them down the barrel until they get above the combustion chamber.
5. Fill combustion chamber with fuel (hairspray) and cover with cap.
6. Point cannon straight up.
7. Prepare stopwatch to time.
8. Fire and start stopwatch.
9. Wait until potato hits the ground.
10. Stop stopwatch upon impact.
11. Record data.
12. Repeat steps for 20 data points.
13. Analyze

Return to Table of Contents

Results

Return to Table of Contents

By use of these following physics formulas:

v = u + at
s = ut + (1/2)at²
Ke = (1/2)mv²
Pe = mgh
We started off by using v = u + at to determine velocity.
By cutting time in half, to get to the point at the top where u = 0, from there we are able to calculate the final velocity using gravity and time.

8.36 seconds total round trip. Divide that in half to get 4.18 seconds.
Set u = 0
Set up equation
v = 0 + (9.8)(4.18)
v = 0 + 40.964
v = 40.964 meters / second

We then used Microsoft Excel to set up a spreadsheet to automatically calculate the velocity for each data point.

Now knowing the velocity, we will be able to calculate the height reached by the potato using s = ut + (1/2)at²

Once again, cutting the time in half to arrive at u = 0
s = (0)(4.18) + (1/2)(9.8)(4.18²)
s = 0 + 85.61475
s = 85.61475 meters

And then setting up a equation in our spreadsheet to calculate height for each data point.

Then using Ke = (1/2)mv² and Pe = mgh to determine energy present, using each formula to check the other.
Ke = (1/2)(.12)(40.964²) = 100.68 Joules
Pe = (.12)(9.8)(85.61) = 100.68 Joules

To account for uncertainty we are including the factors of air friction and the ratio of air to hairspray in the combustion chamber.

Return to Table of Contents

Discussion
When we calculated the actual velocities of the potatoes, we were quite shocked. We were estimating that they would fly around 15 meters/second, but when calculated, it was more like 35-40 m/s. So was our hypothesis conclusive? We think it is. We measured the distance from Daves house to Tonys to be around 105 meters. And when we found the distance our potato could fly, we ended up with 120 meters. We estimated that is would fly at a high rate of speed, we just estimated way low. By use of our physics knowledge, we were able to determine that it flew at a rate of speed unacceptable on Americas freeway system, approximately 90 mph. However it would be accepted on Germanys Autobahn. We also determined that potatoes flying at that high of a velocity tend to make a louder THUD when hitting the ground. Also increasing the crater size left behind. We are currently thinking about researching the interaction between potato mass and velocity on crater size.