The Relationship Between Initial Velocity of a Collision and the Coefficient of Restitution
An investigation by Brian Muhich
Background .:. Hypothesis .:. Method .:. Data .:. Results Discussion .:. Related Websites .:. Bibliography .:. Go Up
Background: .:. Go Up
It is an essential understanding of basic physics that energy is always conserved and cannot be created nor destroyed. When two objects collide, the energy in the system before the collision must equal the energy after the collision. However, the combined kinetic energy of the objects before the collision does not necessarily equal the combined kinetic energy after. In elastic collisions, a collision in which kinetic energy before is equal to kinetic energy after, no energy is lost to the environment. Alternatively, in an inelastic collision, some kinetic energy is lost to the environment resulting in kinetic energy before not equaling kinetic energy after. This change in total kinetic energy is primarily due to this energy being converted to heat and sound, as a result of the deformation of the object.
In the process of all inelastic collisions, the objects involved are deformed during the collision. The following images show a golf ball being hit in slow motion (Titleist YouTube).

During the collision, the ball compresses, and after the collision the ball expands before going back to its original form. These phases of compression and expansion are where the kinetic energy is lost and sound waves are created.
The coefficient of restitution is a value that can be used to evaluate the elasticity of a collision and is equal to the square root of the total kinetic energy after the collision over the total kinetic energy before the collision. A collision with a coefficient of restitution of 1 would be perfectly elastic and would lose no kinetic energy in collisions and a collision with a coefficient of 0 would be completely inelastic and would lose all kinetic energy. The properties of the collision determine the elasticity, one such property being the velocity of the objects involved.
Statement of the Problem:
The purpose of this investigation is to determine the effect that the initial velocity of a collision has on the coefficient of restitution.
Hypothesis: .:. Go Up
I hypothesize that by testing different velocities I will determine that faster moving objects have a higher coefficient of restitution, and therefore, collisions involving higher speeds will resemble elastic collisions and low speed collisions will resemble inelastic collisions. I believe that this will happen because faster collisions cause the object to more quickly snap back to its original shape after being deformed, therefore causing more kinetic energy to be conserved.
(Figure 1)

(Figure 1) For this investigation I used two steel marbles, two sets of photogates, and a ramp/track. The ramp was elevated at a 30° angle and was 30 cm long. I collected data by placing one marble in between the photogates and dropping the other marble from varying heights on the ramp. The first set of photogates measured the initial velocity of the moving marble and the second set of photogates measured the post-collision velocity of the marble that was hit. For this investigation I collected 87 data points, three trials per centimeter difference in length placed up the ramp, starting at the second centimeter mark from the bottom of the ramp.
Equations Used:
Velocity = Distance / Time
Kinetic energy = (1/2)(Mass)(Velocity)^2
Coefficient of Restitution = ((KE post collision)^1/2)/((KE pre collision)^1/2)
(Figure 2)
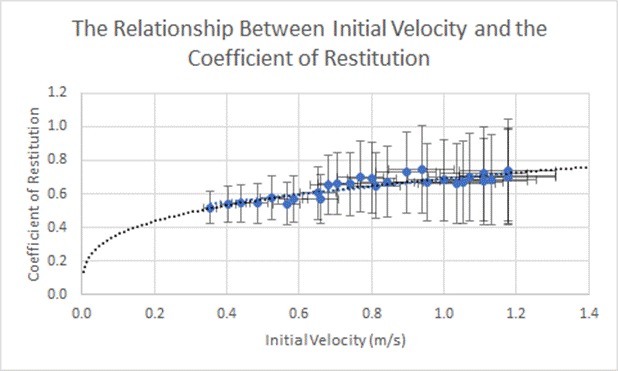
Data Synopsis:
(Figure 2) The coefficient of restitution increases as the initial velocity increases. The trend line of the coefficient of restitution graphed against initial velocity resembles a square root function and further demonstrates a positive correlation. The absolute error of initial velocity and coefficient of restitution increases in later trials, primarily due to rounding higher initial velocities.
Results Discussion: .:. Go Up
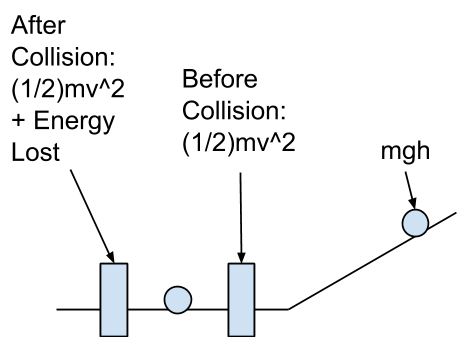
In this system, energy is converted from gravitational potential to kinetic energy, then the collision occurs, in which some kinetic energy is lost due to the deformation of the steel marbles, creating sound and thermal energy.
mgh = (1/2)mv^2 = (1/2)mv^2 + energy lost
m = mass, g = gravity, h = height, v = velocity
(Figure 3)
Based on the positive correlation between the initial velocity and the coefficient of restitution, I can determine that faster collisions more closely resemble elastic collisions than slower collisions. The data I collected in my experiment supports my hypothesis that less energy is lost in a collision involving fast moving objects, therefore making these collisions more elastic. This means that more kinetic energy is transferred from the initial object to the secondary object and less energy is lost to the environment.
The percent error of the data in this experiment grows as the initial velocity increases. This is because the limit of three significant figures collected when gathering data means that as the times recorded by the photogates decrease, a greater percentage of the values is rounded off. Despite the larger percent errors, the trend of positive correlation with the coefficient of restitution and initial velocity remains apparent and valid.
This experiment could be improved with better equipment, such as a air track to eliminate friction and a machine based system
to drop the steel marbles at a more consistent height and time. In addition to
reducing sources of error, this experiment could be improved by collecting more
data from a wider range of initial velocities. A different experiment that
could be conducted in order to determine collision elasticity would be to use a
high speed camera to observe these collisions. By timing how long the steel
marbles remain in a deformed state, comparing these times should demonstrate
different levels of collision elasticity. Finally, one could test if the
pattern of positive correlation between the coefficient of restitution and
initial velocity remains with objects of different densities and hardness.
Related Websites: .:. Go Up
https://hypertextbook.com/facts/2006/restitution.shtml
- A similar study investigating the coefficient of restitution of various balls
www.scienceabc.com/pure-sciences/coefficient-of-restitution-definition-explanation-and-formula.html - An article explaining the coefficient of restitution
www.khanacademy.org/science/physics/linear-momentum/elastic-and-inelastic-collisions/a/what-are-elastic-and-inelastic-collisions
- An article distinguishing between elastic and inelastic collisions
https://www.youtube.com/watch?v=u_22cqNQuo0
- A video explaining how to calculate the coefficient of restitution
https://ipfs.io/ipfs/QmXoypizjW3WknFiJnKLwHCnL72vedxjQkDDP1mXWo6uco/wiki/Coefficient_of_restitution.html
- An article showed the derivation of the formula for finding the coefficient
of restitution
Bibliography: .:. Go Up
Anrica, Deb. “Forms of Energy: Motion, Heat, Light, Sound.” BURN an Energy Journal, 29 Nov. 2015, burnanenergyjournal.com/forms-of-energy-motion-heat-light-sound-2/.
Ashish. “Coefficient Of Restitution: Definition, Explanation And Formula » Science ABC.” Science ABC, Science ABC, 17 Oct. 2017, www.scienceabc.com/pure-sciences/coefficient-of-restitution-definition-explanation-and-formula.html.
Titleist. YouTube, YouTube, 24 Oct. 2013, www.youtube.com/watch?v=6TA1s1oNpbk.
“What Are Elastic and Inelastic Collisions?” Khan Academy, www.khanacademy.org/science/physics/linear-momentum/elastic-and-inelastic-collisions/a/what-are-elastic-and-inelastic-collisions.