Elijah Shumway
Catherine Hancock
Michael Wood
B.I.G.A.S.S.C.A.N.N.O.N. | Introduction | Method | Materials | Data Collection | Diagrams | Results | Data | Errors | Conclusion | Sources | Links | Back to Research |
Introduction
Based upon the original gunpowder cannons, air cannons are a modern twist on the traditional design. Using pneumatic air pressure rather than combustible components, air cannons can be used to fire projectiles at high speeds without the use of explosives or other combustors. The air cannons history is fairly short, used mostly by hobbyists to fire projectiles for fun. In example, a major use of these cannons is for events like Pumpkin-Chunkin, in which people build massive pneumatic cannons to fire pumpkins over vast distances. However, air cannons also have uses in scientific experiments. Due to the nature of the cannon’s design, it is easy to control the exit velocity and force upon a projectile coming out of the barrel. This allows for controlled and repeatable velocities.
The air cannon’s firing mechanic is fairly simple; the system is composed of only three basic parts. First is the pressurized chamber: this is where pressure is built up and stored in order to create the force used to launch the projectile out of the barrel. This piece of the cannon has to be the strongest and one hundred percent air-tight, as it will be dealing with high pressures depending on the user’s aims. If the chamber is not built strong enough, there is a potential that the cannon could burst. The second piece of the system is a valve. It is used to quickly release the pressure from the pressurized chamber into the barrel, creating the force needed to launch the projectile. For the best results, the valve needs to open extremely quickly so the air can rush out in the shortest amount of time. This allows for the strongest force out of the barrel of the cannon. Finally, the last part of the design is the barrel. The barrel is usually much smaller than the pressurized chamber in order to increase the exit velocity due to Bernoulli’s principle and flow rates. The barrel’s other purpose is to ensure that the projectile will fly straight after being fired from the cannon.
The purpose of this investigation is to determine how the variation in pressure within a pressurized air tank affects the exit velocity of an air pressure cannon. Our independent variable in this experiment is the internal pressure of the air cannon; we are able to adjust the pressure up to 100 PSI with an uncertainty of one pound per square inch. Impacted by the differing levels of pressure, the velocity of the tennis ball is our dependent variable and what we are attempting to calculate through our tests.
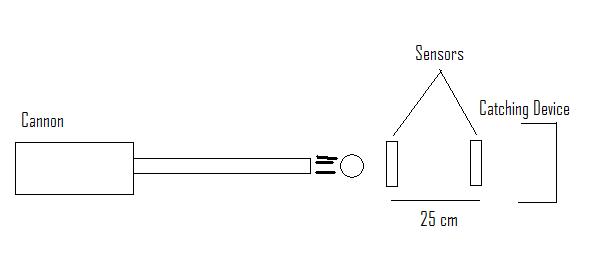
In our tests, we would release the air from the first chamber into the second, launching the ball. After releasing the tennis ball from the cannon, the ball will fly past two Photogate sensors. When the ball passed a sensor, it would block it, starting a timer. It then blocks the second Photogate 25 cm away from the first, triggering the LoggerPro program to start a second timer. We then subtract the initial time from the final time, giving us the elapsed time between the first sensor being blocked and the second. We are then able to calculate the velocity by using the formula V=S/T. We believe that the higher the internal pressure, the faster the air will release from the barrel of the cannon, in turn affecting the velocity of a projectile. The velocity will increase because with a higher pressure there is a higher force behind the ball when it is released.
Air cannons are a fun and effective way to display some aspects of physics, such as pressure and velocity. We decided to build an air-pressure cannon that would shoot tennis balls through Photogate sensors and using LoggerPro software to find the velocity of the ball. Couplings, bushings, various sized PVC pipes, a clean out plug, and a ball valve were the parts used for the air cannon. To hold the cannon in place while shooting, we utilized many clamps, two weights, and some wood. This allowed us to keep the cannon rooted in the same spot while still being able to easily adjust the cannon up and down. In order to fill the chamber of the cannon with pressurized air, we had a power inflator. This power inflator had a max capacity of 150 PSI, but we found that at above 100 PSI, the pump would start to struggle to continue to condense air and the pump hose would pop off the pump due to the high pressure. To gather data on exit velocity, we utilized two Photogate Sensors that were both placed in a wood stand and the Vernier Photogate LoggerPro Software. The sensors were placed 25 cm away from each other, set into a sturdy wood base. The full specific list of materials follows below:
3” Couplings (2)
3” to 1 ½” Bushings (2)
1 ½” PVC Pipe
3” PVC Pipe
4” PVC Pipe back to top
4” Clean Out Plug
1 ½” Ball Valve
Pipe Glue
Photogate Sensors (2)
Vernier Photogate LoggerPro Software
Ryobi Power Inflator
Tennis Balls (a lot)
Wood (various types)
Clamps (3)
Weights (20 lbs to hold cannon base in place)
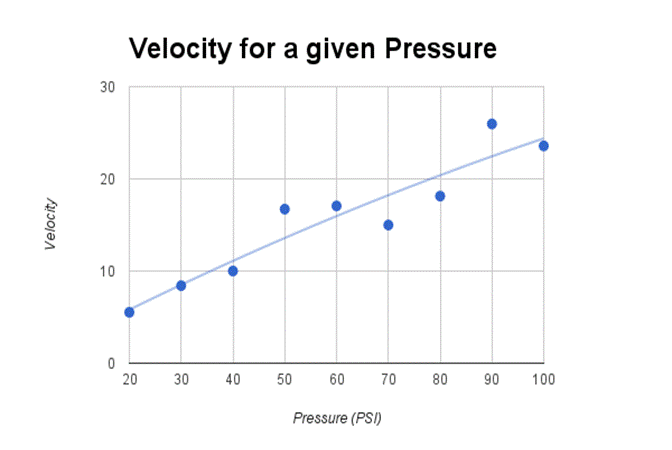
We gathered data by first taking note of each level of pressure we pumped the cannon up to. We started at 20 PSI and made our way up to 100 PSI, testing each level three separate times in order to get a more accurate representation of data. By rapidly opening the valve, the ball would fire out and we would begin our calculations. Firstly, the ball would pass through the two Photogates, calculating the time that it took the ball to pass through one and then the other. Using that gathered time, the LoggerPro software would calculate the velocity by dividing the set distance of 25 centimeters by the time it took to pass through both sensors. With this data, we could see how the variations in pressure affected the exit velocity of the tennis ball.
We decided to put the photogates close to the cannon’s barrel in order to measure the velocity of the ball as soon as it comes out. This minimized our error by not allowing air resistance to slow the ball much before measuring its velocity. We also had a bin set up at the end of the barrel with a cloth shield that would wrap around the ball as it hit so the ball would not ricochet back or damage any piece of the set up.
Diagrams: back to top


Data back to top
Our experimental uncertainty mainly came from the variable speed with which the cannon’s valve was opened. We had the same person open it for each trial, but they, being human, could not be expected to open the valve at the exact same rate every time. This may have caused the ball to be launched at differing velocities, thereby leading to large error. Another source of error is the fact that the ball would sometimes graze the photogates as it flew through them. This was because they were barely tall enough for the tennis ball to fit, so any slight deviation in the barrel would cause the ball to run into them. Our timing may have been skewed by this, but it also caused us to have to reset the position of the photogates after each trial, possibly causing a small amount of error from trial to trial. Finally, though the wadding for the cannon was the same throughout all of the trials, the tightness of the packing may have varied test from test due to the nature of the wadding, perhaps making the ball behave differently while in flight.
Conclusion:
back to top
The data did support our hypothesis that with more pressure comes faster velocities; however, it is hard to say what kind of relationship is present. The formula for the line of best fit seems to indicate a logarithmic relation between pressure and velocity, but our data is not clear enough to draw any definite conclusion on the matter. An argument could be made for both a linear and a quadratic relationship with the data we gathered. What is clear is that it does indeed trend upwards. Our additional hypothesis is that there is a quadratic relation between pressure and velocity. To further research this topic, we would definitely invest in a solenoid valve in order to mitigate our largest source of error. A solenoid would remove the uncertainty in the time that it took for the air to release, and would make each individual test of a PSI more accurate and controlled. We would also include more intervals, perhaps every 5 PSI instead of 10. This way, we would have more data points in order to draw conclusions from. Lastly, we would come up with a more precise way of measuring the ball’s velocity, like fixing the gates in place better, or simply using something the ball would not run into. All in all, this experiment was a lot of fun, and it taught us about important scientific principles.
"Air Cannon Models." Air Cannons. Air Cannons, Inc., n.d. Web.
"Bernoulli's Equation." Princeton University. The Trustees of Princeton University, n.d. Web.
"Flow in Pipe - Bernoulli Equation." Pipe Flow Calculations. Pipe Flow Calculations, n.d. Web.
Schupak, Amanda. "How It Works: The Artillery-Grade 600 MPH Pumpkin Cannon." Popular Science. Bonnier Corporation Company, 25 Jan. 2010. Web.
Wise, Edwin. "Boom Stick: The High Powered PVC Air Cannon | Make:." Make:. Maker Media, Inc., 20 Nov. 2015. Web.
https://www.youtube.com/watch?v=-W6gzG7ZwGk
: World’s fastest Punkin Chunker showing the velocitive power of air
https://www.youtube.com/watch?v=SdaetY_dZ0s
: Shooting a pumpkin through a trailer with air power showing the destructive
power of air
https://www.youtube.com/watch?v=eKMKtpaq1gE
: Air rifle showing the useful power of air
http://www.iontrap.wabash.edu/adlab/papers/S2011_Buresh_Rohrbach_air_cannon.pdf
: What we did, but so, so, so, so, so much better at showing the educational
power of air
http://tuhsphysics.ttsd.k12.or.us/Research/IB07/GramRecoSire/index.htm
: Somebody else’s lab, you decide which is better. You know which one it is.
You Know… showing a comparison of the power of air